Advertisements
Chapters
![Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board chapter 6 - वृत्त - Shaalaa.com](/images/geometry-mathematics-2-hindi-9-standard-maharashtra-state-board_6:2d35025042714886a119651abfd86519.png "Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board chapter 6 - वृत्त")
Advertisements
Solutions for Chapter 6: वृत्त
Below listed, you can find solutions for Chapter 6 of Maharashtra State Board Balbharati for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board.
Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board 6 वृत्त प्रश्नसंग्रह 6.1 [Page 79]
वृत्त के केंद्र O से 8 सेमी की दूरी पर जीवा AB स्थित है। जीवा AB की लंबाई 12 सेमी है तो वृत्त का व्यास ज्ञात कीजिए।
किसी वृत्त का व्यास 26 सेमी तथा जीवा की लंबाई 24 सेमी है तो वह जीवा वृत्त के केंद्र से कितनी दूरी पर होगी?
34 सेमी त्रिज्यावाले वृत्त की एक जीवा केंद्र से 30 सेमी की दूरी पर हो तो जीवा की लंबाई ज्ञात कीजिए।
O केंद्रवाले वृत्त की त्रिज्या 41 सेमी है। वृत्त की जीवा PQ की लंबाई 80 सेमी हो तो जीवा PQ की केंद्र से दूरी ज्ञात कीजिए।
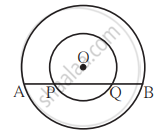
आकृति में बिंदु O केंद्रवाले दो वृत्त हैं। बड़े वृत्त की जीवा AB यह जीवा छोटे वृत्त के बिंदु P तथा Q पर प्रतिच्छेदित करती है। सिद्ध कीजिए कि AP = BQ
सिद्ध कीजिए कि यदि वृत्त का व्यास दो जीवाओं को समद्विभाजित करता हो तो वे जीवाएँ परस्पर समांतर होती हैं।
Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board 6 वृत्त प्रश्नसंग्रह 6.2 [Page 82]
किसी वृत्त की त्रिज्या 10 सेमी है। उस वृत्त में 16 सेमी लंबाईवाली दो जीवाएँ हैं तो उन जीवाओं की केंद्र से दूरी ज्ञात कीजिए?
एक वृत्त में समान लंबाईवाली दो जीवाएँ हैं। वे जीवाएँ केंद्र से 5 सेमी दूरी पर है। वृत्त की त्रिज्या 13 सेमी है तो जीवाओं की लंबाई ज्ञात कीजिए।
C केंद्रवाले वृत्त में रेख PM तथा रेख PN सर्वांगसम जीवाएँ है तो सिद्ध कीजिए किरण PC यह `angle`NPM की समद्विभाजक है।
Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board 6 वृत्त प्रश्नसंग्रह 6.3 [Page 86]
ΔABC के अंतर्वृत्त की रचना कीजिए, जिसमें `angle`B =100°, BC = 6.4 सेमी `angle`C = 50°।
ΔPQR के परिवृत्त की रचना कीजिए, जिसमें `angle`P = 70°, `angle`R = 50°, QR = 7.3 सेमी।
ΔXYZ के अंतर्वृत्त की रचना कीजिए, जिसमें XY = 6.7 सेमी, YZ = 5.8 सेमी, XZ = 6.9 सेमी।
ΔLMN में LM = 7.2 सेमी, `angle`M = 105°, MN = 6.4 सेमी तो ΔLMN परिवृत्त की रचना कीजिए।
ΔDEF के परिवृत्त की रचना कीजिए, जिसमें DE = EF = 6 सेमी `angle`F = 45°।
Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board 6 वृत्त प्रकीर्ण प्रश्नसंग्रह 6 [Pages 86 - 87]
निम्नलिखित बहु वैकल्पिक प्रश्नों के दिए गए उत्तरों में से सही विकल्प चुनिए।
एक वृत्त की त्रिज्या 10 सेमी तथा जीवा की केंद्र से दूरी 6 सेमी है तो उस जीवा की लंबाई कितनी होगी?
16 सेमी
8 सेमी
12 सेमी
32 सेमी
त्रिभुज के तीनों कोणों के समद्विभाजक संगामी होते हैं। उनके संगामी बिंदु को क्या कहते हैं?
माध्यिका संगम
परिकेंद
अंतः केंद्र
लंब केंद
त्रिभुज के सभी शीर्षबिंदुओं से जाने वाले वृत्त को क्या कहते हैं?
परिवृत्त
अंतःवृत्त
सर्वांगसम वृत्त
एक केंद्रीय वृत्त
किसी वृत्त की जीवा की लंबाई 24 सेमी तथा केंद्र से जीवा 5 सेमी दूरी पर है तो वृत्त की त्रिज्या ज्ञात कीजिए?
12 सेमी
13 सेमी
14 सेमी
15 सेमी
2.9 सेमी त्रिज्यावाले वृत्त की सबसे बड़ी जीवा की लंबाई कितनी हो सकती है?
3.5 सेमी
7 सेमी
10 सेमी
5.8 सेमी
किसी O केंद्रवाले वृत्त की त्रिज्या 4 सेमी है। l(OP) = 4.2 सेमी हो तो बिंदु ‘P’ कहाँ होगा?
केंद्र पर
वृत्त के अंतःभाग में
वृत्त के बहिर्भाग में
वृत्त पर
किसी वृत्त की समांतर जीवाओं की लंबाई क्रमशः 6 सेमी तथा 8 सेमी है। उस वृत्त की त्रिज्या 5 सेमी हो तो उन जीवाओं के बीच दूरी कितनी होगी?
2 सेमी
1 सेमी
8 सेमी
7 सेमी
समबाहु त्रिभुज ΔDSP में DS = 7.5 सेमी तो ΔDSP केपरिवृत्त तथा अंतर्वृत्त की रचना कीजिए।परिवृत्त तथा अंतर्वृत्त की त्रिज्या का अनुपात ज्ञात कीजिए।
ΔNTS मेंपरिवृत्त तथा अंतर्वृत्त की रचना कीजिए जिसमें NT = 5.7 सेमी, TS = 7.5 सेमी `angle`NTS = 110°
आकृति में C वृत्त का केंद्र है। रेख QT व्यास है। CT = 13, CP = 5 हो तो जीवा RS ज्ञात कीजिए।
आकृति में P वृत्त का केंद्र है। जीवा AB तथा जीवा CD परस्पर व्यास के बिंदु E पर प्रतिच्छेदित करते हैं। यदि `angle`AEP ≅ `angle`DEP तो सिद्ध कीजिए कि AB = CD
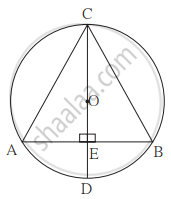
आकृति में O केंद्रवालेवृत्त का व्यास CD तथा जीवा AB है। व्यास CD जीवा AB के बिंदु E पर लंब है तो सिद्ध कीजिए कि ΔABC समद्विबाहु त्रिभुज है।
Solutions for 6: वृत्त
Balbharati solutions for Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board chapter 6 - वृत्त
Shaalaa.com has the Maharashtra State Board Mathematics Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board Maharashtra State Board solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Balbharati solutions for Mathematics Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board Maharashtra State Board 6 (वृत्त) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Balbharati textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board chapter 6 वृत्त are वृत्त - केंद्र, त्रिज्या, व्यास, जीवा, त्रिज्यखंड, वृत्तखंड, अर्धवृत्त, परिधि, चाप, अभ्यंतर और बहिर्भाग, संकेंद्रित वृत्त, वृत्त की जीवा के गुणधर्म, प्रमेय - वृत्त के केंद्र से जीवा पर डाला गया लंब उस जीवा को समद्विभाजित करता है।, प्रमेय - वृत्त केंद्र तथा जीवा के मध्यबिंदु को जोड़ने वाला रेखाखंड जीवा पर लंब होता है।, वृत्त की सर्वांगसम जीवाओं तथा उनके केंद्र से दूरी संबंधी गुणधर्म, सर्वांगसम जीवाओं के गुणधर्म, प्रमेय - एक ही वृत्त की सर्वांगसम जीवाएँ केंद से समदूरस्थ होती हैं।, प्रमेय - एक ही वृत्त की केंद्र से समदूरस्थ जीवाएँ सर्वांगसम होती हैं।, त्रिभुज का अंतर्वृत्त, त्रिभुज के अंतर्वृत्त की रचना करना, त्रिभुज का परिवृत्त, त्रिभुज के परिवृत्त की रचना करना.
Using Balbharati Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board solutions वृत्त exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Balbharati Solutions are essential questions that can be asked in the final exam. Maximum Maharashtra State Board Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board students prefer Balbharati Textbook Solutions to score more in exams.
Get the free view of Chapter 6, वृत्त Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board additional questions for Mathematics Geometry (Mathematics 2) [Hindi] 9 Standard Maharashtra State Board Maharashtra State Board, and you can use Shaalaa.com to keep it handy for your exam preparation.
