Advertisements
Advertisements
प्रश्न
A block of mass m is placed on a triangular block of mass M which in turn is placed on a horizontal surface as shown in figure. Assuming frictionless surfaces find the velocity of the triangular block when the smaller block reaches the bottom end.

उत्तर
According to the question, the surface is frictionless. Thus, the block m will slide down the inclined plane of mass M.
Acceleration, a1 = g sin α (Relative to the inclined plane)
The horizontal component of acceleration a1 is given by ax = g sin α cos α, for which the block M accelerates towards left.
Let the left acceleration be a2.
By the concept of centre of mass, we can say that the external force is zero in the horizontal direction.
\[m a_x = (M + m) a_2\]
Absolute (resultant) acceleration of m on the plane M, along the direction of the incline will be = \[a = g \sin \alpha - a_2 \cos \alpha\]
Let the time taken by the block m to reach the bottom end be t.
Now,
\[s = ut + \left( \frac{1}{2} \right)a t^2 \]
\[ \Rightarrow \frac{h}{\sin \alpha} = \left( \frac{1}{2} \right)a t^2 \]
\[ \Rightarrow t = \sqrt{\frac{2h}{a \sin \alpha}}\]
Thus, the velocity of the bigger block after time t will be,
\[v_m = u = a_2 t\]
\[ = \frac{mg \sin \alpha \cos \alpha}{M + m} \sqrt{\frac{2h}{a \sin \alpha}} = \left[ \frac{2 m^2 g^2 h \sin^2 \alpha \cos^2 \alpha}{(M + m )^2 a \sin \alpha} \right]^{1/2}\]
Subtracting the value of a from equation (2), we get:
\[v_M = \left[ \frac{2 m^2 g^2 h \sin^2 \alpha}{(M + m )^2 \sin \alpha} \times \frac{\cos^2 \alpha}{g \sin \alpha} \frac{(M + m)}{(M + m \sin^2 \alpha)} \right]\]
APPEARS IN
संबंधित प्रश्न
Give the location of the centre of mass of a
- sphere,
- cylinder,
- ring, and
- cube,
each of uniform mass density. Does the centre of mass of a body necessarily lie inside the body?
In the HCl molecule, the separation between the nuclei of the two atoms is about 1.27 Å (1 Å = 10–10 m). Find the approximate location of the CM of the molecule, given that a chlorine atom is about 35.5 times as massive as a hydrogen atom and nearly all the mass of an atom is concentrated in its nucleus.
A child sits stationary at one end of a long trolley moving uniformly with a speed V on a smooth horizontal floor. If the child gets up and runs about on the trolley in any manner, what is the speed of the CM of the (trolley + child) system?
The centre of mass is defined as \[\vec{R} = \frac{1}{M} \sum_i m_i \vec{r_i}\]. Suppose we define "centre of charge" as \[\vec{R}_c = \frac{1}{Q} \sum_i q_i \vec{r_i}\] where qi represents the ith charge placed at \[\vec{r}_i\] and Q is the total charge of the system.
(a) Can the centre of charge of a two-charge system be outside the line segment joining the charges?
(b) If all the charges of a system are in X-Y plane, is it necessary that the centre of charge be in X-Y plane?
(c) If all the charges of a system lie in a cube, is it necessary that the centre of charge be in the cube?
You are waiting for a train on a railway platform. Your three-year-old niece is standing on your iron trunk containing the luggage. Why does the trunk not recoil as she jumps off on the platform?
If the external force acting on a system have zero resultant, the centre of mass
(a) must not move
(b) must not accelerate
(c) may move
(d) may accelerate.
A nonzero external force acts on a system of particles. The velocity and the acceleration of the centre of mass are found to be v0 and a0 at instant t. It is possible that
(a) v0 = 0, a0 = 0
(b) v0 = 0, a0 ≠ 0
(c) v0 ≠ 0, a0 = 0
(d) v0 ≠ 0, a0 ≠ 0
Three particles of masses 1.0 kg, 2.0 kg and 3.0 kg are placed at the corners A, B and C respectively of an equilateral triangle ABC of edge 1 m. Locate the centre of mass of the system.
Find the ratio of the linear momenta of two particles of masses 1.0 kg and 4.0 kg if their kinetic energies are equal.
Two fat astronauts each of mass 120 kg are travelling in a closed spaceship moving at a speed of 15 km/s in the outer space far removed from all other material objects. The total mass of the spaceship and its contents including the astronauts is 660 kg. If the astronauts do slimming exercise and thereby reduce their masses to 90 kg each, with what velocity will the spaceship move?
Solve the following problem.
A uniform solid sphere of radius R has a hole of radius R/2 drilled inside it. One end of the hole is at the center of the sphere while the other is at the boundary. Locate center of mass of the remaining sphere.
A round object of mass M and radius R rolls down without slipping along an inclined plane. The frictional force, ______
Define centre of mass.
The centre of mass of a system of two particles divides the distance between them ______.
A bullet of mass 20 gram is fired from a gun of mass 2.5 kg with a speed of 750 m/s. The magnitude of recoil velocity of the gun is ______.
Which of the following statements are correct?
For which of the following does the centre of mass lie outside the body?
A uniform square plate has a small piece Q of an irregular shape removed and glued to the centre of the plate leaving a hole behind figure. The CM of the plate is now in the following quadrant of x-y plane ______.
A uniform square plate S (side c) and a uniform rectangular plate R (sides b, a) have identical areas and masses (Figure).
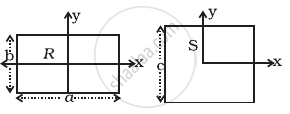
Show that
- IxR/IxS < 1
- IyR/IyS > 1
- IzR/IzS > 1
