Advertisements
Advertisements
प्रश्न
A boy is standing on the ground and flying a kite with 100m of sting at an elevation of 30°. Another boy is standing on the roof of a 10m high building and is flying his kite at an elevation of 45°. Both the boys are on opposite sides of both the kites. Find the length of the string that the second boy must have so that the two kites meet.
उत्तर
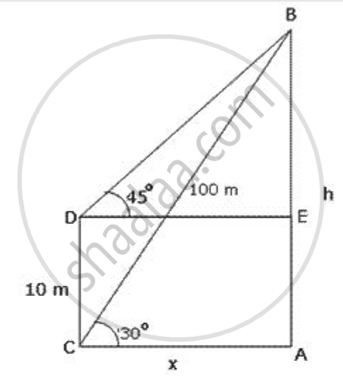
Let C be the position of the first boy and D be the position of the second boy who is standing on the roof of a 10 m high building.
Let B be the position of the kites of both the boys.
Let AB = h and CA = x.
In ΔABC,
sin30° = `"h"/100`
⇒ `1/2 = "h"/100`
⇒ h = 50 ... (1)
In ΔBDE,
`tan45^circ = "BE"/"BD"`
⇒ `1 = ("h" - 10)/"X"`
⇒ X = (h - 10) ...(2)
From (1) and (2),
x = 50 - 10 = 40
In ΔBDE,
`sin45^circ = "BE"/"BD"`
⇒ `1/sqrt(2) = ("h" - 10)/("BC")`
⇒ `"BC" = sqrt(2)(50-10) = 40sqrt(2)`
Thus, the required length of the string that the second boy must have `40sqrt(2)` m
APPEARS IN
संबंधित प्रश्न
A conical tent is to accommodate 77 persons. Each person must have 16 m3 of air to breathe. Given the radius of the tent as 7 m, find the height of the tent and also its curved surface area.
Prove the following identities:
tan2 A – sin2 A = tan2 A . sin2 A
From the top of a cliff 92 m high, the angle of depression of a buoy is 20°. Calculate, to the nearest metre, the distance of the buoy from the foot of the cliff.
From the top of a cliff, 60 metres high, the angles of depression of the top and bottom of a tower are observed to be 30° and 60°. Find the height of the tower.
The radius of a circle is given as 15 cm and chord AB subtends an angle of 131° at the centre C of the circle. Using trigonometry, calculate:
- the length of AB;
- the distance of AB from the centre C.
The top of a palm tree having been broken by the wind struck the ground at an angle of 60° at a distance of 9m from the foot of the tree. Find the original height of the palm tree.
A vertical tow er stands on a horizontal plane and is surmounted by a flagstaff of height 7m. From a point on the plane the angle of elevation of the bottom of the flagstaff is 30° and that of the top of the ft agstaff is 45°. Find the height of the tower.
The horizontal distance between two trees of different heights is 100m. The angle of depression of the top of the first tree when seen from the top of the second tree is 45°. If the height of the second tree is 150m, find the height of the first tree.
If the angle of elevation of a cloud from a point h m above a lake is α, and the angle of depression of its reflection in the lake be β, prove that distance of the cloud from the point of observation is `("2h"secα)/(tanα - tanβ)`.
A man on the top of vertical observation tower observes a car moving at a uniform speed coming directly towards it. If it takes 12 minutes for the angle of depression to change from 30° to 45°, how soon after this will the car reach the observation tower? (Give your answer correct to nearest seconds).
