Advertisements
Advertisements
प्रश्न
If the angle of elevation of a cloud from a point h m above a lake is α, and the angle of depression of its reflection in the lake be β, prove that distance of the cloud from the point of observation is `("2h"secα)/(tanα - tanβ)`.
उत्तर
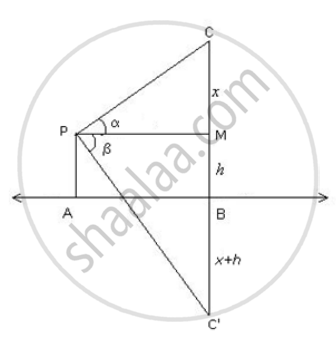
Let AB be the surface of the lake and let P be an point of observation such that AP = h meters . Let c be the position of the cloud and C' be its reflection in the lake . Then ∠CPM = α and ∠MPC = β. Let CM = x.
Then , CB = CM + MB = CM + PA = x + h
In ΔCPM,
tan α = `"CM"/"PM"`
⇒ `tan α = "x"/"AB"` [∵ PM = AB]
⇒ AB = x cotα ...(1)
In ΔPMC',
`tanβ = (C'M)/(PM)`
⇒ `tanβ = ("x" + "2h")/"AB"`
⇒ AB = (x + 2h)cotβ ....(2)
From (1) and (2),
x cotα = (x + 2h) cot β
⇒ `"x"(1/tanα - 1/tanβ) = "2h"/tanβ`
⇒ `"x"((tanβ - tanα) /(tanα tanβ)) = "2h"/tanβ`
⇒ x = `("2h"tanα)/(tanβ- tanα)`
Again, in Δ CPM,
`sin α = "CM"/"PC"` = `"x"/("PC")`
⇒ PC = `"x"/sin α`
⇒ `"PC" = ("2h"tanα)/(tanβ - tanα) xx 1/sin α`
⇒ `"PC" = ("2h"secα)/(tanβ - tanα)`
Hence , the distance of the cloud from the point of observation is `("2h"secα)/(tanβ - tanα)`.
APPEARS IN
संबंधित प्रश्न
The horizontal distance between two towers is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the second tower is 30° and 24° respectively.
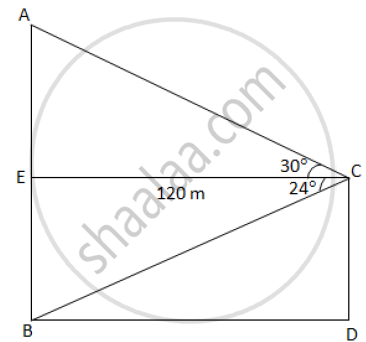
Find the height of the two towers. Give your answer correct to 3 significant figures
Two climbers are at points A and B on a vertical cliff face. To an observer C, 40 m from the foot of the cliff, on the level ground, A is at an elevation of 48° and B of 57°. What is the distance between the climbers?
The angle of elevation of the top of a tower is observed to be 60°. At a point, 30 m vertically above the first point of observation, the elevation is found to be 45°. Find:
- the height of the tower,
- its horizontal distance from the points of observation.
From the top of a cliff, 60 metres high, the angles of depression of the top and bottom of a tower are observed to be 30° and 60°. Find the height of the tower.
The radius of a circle is given as 15 cm and chord AB subtends an angle of 131° at the centre C of the circle. Using trigonometry, calculate:
- the length of AB;
- the distance of AB from the centre C.
From a point, 36 m above the surface of a lake, the angle of elevation of a bird is observed to be 30° and the angle of depression of its image in the water of the lake is observed to be 60°. Find the actual height of the bird above the surface of the lake.
A vertical pole is 90m high and the length of its shadow is `90sqrt(3)`. what is the angle of elevation of the sun ?
Two-person standing on the same side of a tower in a straight line with it measures the angle of elevation of the top of the tower as 25° and 50° respectively. If the height of the tower is 70 m find the distance between the two-person.
An aeroplane at an altitude of 250 m observes the angle of depression of two Boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.
The angles of elevation of the top of a tower from two points A and B at a distance of a and b respectively from the base and in the same straight line with it are complementary. Prove that the height of the tower is `sqrt(ab)`.
