Advertisements
Advertisements
प्रश्न
A narrow beam of light passes through a slab obliquely and is then received by an eye following figure. The index of refraction of the material in the slab fluctuates slowly with time. How will it appear to the eye? The twinkling of stars has a similar explanation.
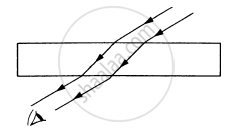
उत्तर
The beam of light will seem to appear and disappear, just like the twinkling of the stars. This is because the refractive index of the material in the slab fluctuates slowly with time. So, the light rays get refracted differently with time and this causes them to get concentrated during certain times, or get diffused at other times.
APPEARS IN
संबंधित प्रश्न
For the same value of angle of incidence, the angles of refraction in three media A, B and C are 15°, 25° and 35° respectively. In which medium would the velocity of light be minimum?
A glass lens of refractive index 1.45 disappears when immersed in a liquid. What is the value of refractive index of the liquid?
Is the formula "Real depth/Apparent depth = μ" valid if viewed from a position quite away from the normal?
A vessel contains water up to a height of 20 cm and above it an oil up to another 20 cm. The refractive indices of the water and the oil are 1.33 and 1.30 respectively. Find the apparent depth of the vessel when viewed from above.
Consider the situation in figure. The bottom of the pot is a reflecting plane mirror, S is a small fish and T is a human eye. Refractive index of water is μ. (a) At what distance(s) from itself will the fish see the image(s) of the eye? (b) At what distance(s) from itself will the eye see the image(s) of the fish.

A convex lens of focal length 20 cm and a concave lens of focal length 10 cm are placed 10 cm apart with their principal axes coinciding. A beam of light travelling parallel to the principal axis and having a beam diameter 5.0 mm, is incident on the combination. Show that the emergent beam is parallel to the incident one. Find the beam diameter of the emergent beam.
A ray of light travelling in a transparent medium of refractive index n falls, on a surface separating the medium from air at an angle of incidents of 45°. The ray can undergo total internal reflection for the following n.
Obtain the equation for apparent depth.
What is critical angle and total internal reflection?
Obtain the equation for critical angle.
What is looming?
What is Snell’s window?
How does an endoscope work?
Obtain the equation for radius of illumination (or) Snell’s window.
Light travels from an optically denser medium 'A' into the optically rarer medium 'B' with speeds 1.8 × 108 m/s and 2.7 × 108 m/s respectively. Then critical angle between them is ______.
(µ1 and µ2 are the refractive indices of media A and B respectively.)
Light travels in two media A and B with speeds 1.8 × 108 ms−1 and 2.4 × 108 ms−1 respectively. Then the critical angle between them is:
A ray of unpolarised light is incident on the surface of glass plate of µ = 1.54 at polarising angle, then angle of refraction is
Show that for a material with refractive index `µ ≥ sqrt(2)`, light incident at any angle shall be guided along a length perpendicular to the incident face.
A beam of light travels from air into a medium. Its speed and wavelength in the medium are 1.5 × 108 ms-1 and 230 nm respectively. The wavelength of light in the air will be ______.
Using Huygen's wave theory, show that (for refraction of light):
`sin i/sin r = "constant"`
where terms have their usual meaning. You must draw a neat and labelled diagram.
