Advertisements
Advertisements
प्रश्न
A transparent paper (refractive index = 1.45) of thickness 0.02 mm is pasted on one of the slits of a Young's double slit experiment which uses monochromatic light of wavelength 620 nm. How many fringes will cross through the centre if the paper is removed?
उत्तर
Given:-
Refractive index of the paper, μ = 1.45
The thickness of the plate,
\[t = 0 . 02 mm = 0 . 02 \times {10}^{- 3} m\]
Wavelength of the light,
\[\lambda = 620 nm = 620 \times {10}^{- 9} m\]
We know that when we paste a transparent paper in front of one of the slits, then the optical path changes by \[\left( \mu - 1 \right)t.\]
And optical path should be changed by λ for the shift of one fringe.
∴ Number of fringes crossing through the centre is
\[n = \frac{\left( \mu - 1 \right)t}{\lambda}\]
\[ = \frac{\left( 1 . 45 - 1 \right) \times 0 . 02 \times {10}^{- 3}}{620 \times {10}^{- 9}}\]
\[ = 14 . 5\]
Hence, 14.5 fringes will cross through the centre if the paper is removed.
APPEARS IN
संबंधित प्रश्न
In Young' s experiment the ratio of intensity at the maxima and minima . in the interference pattern is 36 : 16. What is the ratio of the widths of the two slits?
The intensity at the central maxima in Young’s double slit experiment is I0. Find out the intensity at a point where the path difference is` lambda/6,lambda/4 and lambda/3.`
A beam of light consisting of two wavelengths, 650 nm and 520 nm, is used to obtain interference fringes in a Young’s double-slit experiment.
What is the least distance from the central maximum where the bright fringes due to both the wavelengths coincide?
Write two characteristics features distinguish the diffractions pattern from the interference fringes obtained in Young’s double slit experiment.
Can we perform Young's double slit experiment with sound waves? To get a reasonable "fringe pattern", what should be the order of separation between the slits? How can the bright fringes and the dark fringes be detected in this case?
In a Young's double slit experiment, two narrow vertical slits placed 0.800 mm apart are illuminated by the same source of yellow light of wavelength 589 nm. How far are the adjacent bright bands in the interference pattern observed on a screen 2.00 m away?
A double slit S1 − S2 is illuminated by a coherent light of wavelength \[\lambda.\] The slits are separated by a distance d. A plane mirror is placed in front of the double slit at a distance D1 from it and a screen ∑ is placed behind the double slit at a distance D2 from it (see the following figure). The screen ∑ receives only the light reflected by the mirror. Find the fringe-width of the interference pattern on the screen.
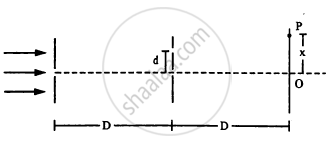
Consider the arrangement shown in the figure. The distance D is large compared to the separation d between the slits.
- Find the minimum value of d so that there is a dark fringe at O.
- Suppose d has this value. Find the distance x at which the next bright fringe is formed.
- Find the fringe-width.
Consider the arrangement shown in the figure. By some mechanism, the separation between the slits S3 and S4 can be changed. The intensity is measured at the point P, which is at the common perpendicular bisector of S1S2 and S2S4. When \[z = \frac{D\lambda}{2d},\] the intensity measured at P is I. Find the intensity when z is equal to
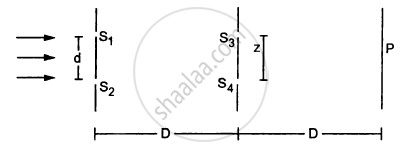
(a) \[\frac{D\lambda}{d}\]
(b) \[\frac{3D\lambda}{2d}\] and
(c) \[\frac{2D\lambda}{d}\]
Draw a neat labelled diagram of Young’s Double Slit experiment. Show that `beta = (lambdaD)/d` , where the terms have their usual meanings (either for bright or dark fringe).
When a beam of light is used to determine the position of an object, the maximum accuracy is achieved, if the light is ______.
In Young’s double slit experiment, what is the effect on fringe pattern if the slits are brought closer to each other?
An unpolarised beam of intensity 2a2 passes through a thin polaroid. Assuming zero absorption in the polaroid, the intensity of emergent plane polarised light will be
Two slits, 4mm apart, are illuminated by light of wavelength 6000 A° what will be the fringe width on a screen placed 2 m from the slits?
In a Young’s double slit experiment, the source is white light. One of the holes is covered by a red filter and another by a blue filter. In this case ______.
How will the interference pattern in Young's double-slit experiment be affected if the screen is moved away from the plane of the slits?
In Young's double slit experiment using light of wavelength 600 nm, the slit separation is 0.8 mm and the screen is kept 1.6 m from the plane of the slits. Calculate
- the fringe width
- the distance of (a) third minimum and (b) fifth maximum, from the central maximum.
In Young's double-slit experiment, the screen is moved away from the plane of the slits. What will be its effect on the following?
- The angular separation of the fringes.
- Fringe-width.
