Advertisements
Advertisements
प्रश्न
A triangle and a parallelogram have the same base and the same area. If the sides of triangle are 26 cm, 28 cm and 30 cm, and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
उत्तर
For triangle
Perimeter of triangle = (26 + 28 + 30) cm = 84 cm
2s = 84 cm
s = 42 cm
By Heron’s formula,
`"Area of triangle "=sqrt(s(s-a)(s-b)(s-c))`
`"Area of triangle "=[sqrt(42(42-26)(42-28)(42-30))]cm^2`
`=[sqrt(42(16)(14)(12))]cm^2`
= 336 cm2
Let the height of the parallelogram be h.
Area of parallelogram = Area of triangle
h × 28 cm = 336 cm2
h = 12 cm
Therefore, the height of the parallelogram is 12 cm.
APPEARS IN
संबंधित प्रश्न
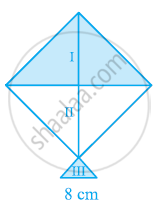
A kite in the shape of a square with a diagonal 32 cm and an isosceles triangles of base 8 cm and sides 6 cm each is to be made of three different shades as shown in the given figure. How much paper of each shade has been used in it?
The sides of a quadrilateral, taken in order are 5, 12, 14 and 15 meters respectively, and the angle contained by the first two sides is a right angle. Find its are
Find the area of a quadrilateral ABCD in which AD = 24 cm, ∠BAD = 90° and BCD forms an equilateral triangle whose each side is equal to 26 cm. (Take √3 = 1.73)
Find the area of an equilateral triangle having each side 4 cm.
If each side of a triangle is doubled, the find percentage increase in its area.
If each side of a equilateral triangle is tripled then what is the percentage increase in the area of the triangle?
The sides of a triangle are 11 cm, 15 cm and 16 cm. The altitude to the largest side is
A square and an equilateral triangle have equal perimeters. If the diagonal of the square is \[12\sqrt{2}\] cm, then area of the triangle is
The sides of a triangle are 35 cm, 54 cm and 61 cm, respectively. The length of its longest altitude ______.
The area of a regular hexagon of side ‘a’ is the sum of the areas of the five equilateral triangles with side a.
