Advertisements
Advertisements
प्रश्न
The sides of a quadrilateral, taken in order are 5, 12, 14 and 15 meters respectively, and the angle contained by the first two sides is a right angle. Find its are
उत्तर
Given that sides of quadrilateral are AB = 5 m, BC = 12 m, CD = 14 m and DA = 15 m
AB = 5m, BC = 12m, CD = 14 m and DA = 15 m
Join AC
Area of ΔABC = `1/2`×𝐴𝐵×𝐵𝐶 [∵𝐴𝑟𝑒𝑎 𝑜𝑓 Δ𝑙𝑒=`1/2`(3𝑥+1)]
= `1/2×5×12`
= 30 cm2
In ΔABC By applying Pythagoras theorem.
`AC^2=AB^2+BC^2`
`⇒AC=sqrt(5^2+12^2)`
`⇒sqrt(25+144)`
`⇒sqrt169=13m`
𝑁𝑜𝑤 𝑖𝑛 Δ𝐴𝐷𝐶
Let 2s be the perimeter

∴ 2s = (AD + DC + AC)
⇒ S = `1/2`(15+14+13)=`1/2`×42=21𝑚
By using Heron’s formula
∴ Area of ΔADC = `sqrt(S(S-AD)(S-DC)(S-AC))`
`=sqrt(21(21-15)(21-14)(21-13))`
`sqrt(21xx6xx7xx8)`
∴𝐴𝑟𝑒𝑎 𝑜𝑓 𝑞𝑢𝑎𝑑𝑟𝑖𝑙𝑎𝑡𝑒𝑟𝑎𝑙 𝐴𝐵𝐶𝐷=𝑎𝑟𝑒𝑎 𝑜𝑓 (Δ𝐴𝐵𝐶)+𝐴𝑟𝑒𝑎 𝑜𝑓 (Δ𝐴𝐷𝐶) = 30 + 84 = `114 m^2`
APPEARS IN
संबंधित प्रश्न
Find the area of a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.
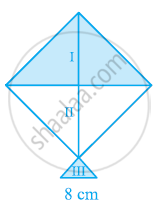
A kite in the shape of a square with a diagonal 32 cm and an isosceles triangles of base 8 cm and sides 6 cm each is to be made of three different shades as shown in the given figure. How much paper of each shade has been used in it?
Two parallel side of a trapezium are 60 cm and 77 cm and other sides are 25 cm and 26 cm. Find the area of the trapezium.
Find the area of a rhombus whose perimeter is 80 m and one of whose diagonal is 24 m.
Find the area of an equilateral triangle having each side 4 cm.
The perimeter of a triangullar field is 144 m and the ratio of the sides is 3 : 4 : 5. Find the area of the field.
The base of an isosceles right triangle is 30 cm. Its area is
The sides of a triangular field are 325 m, 300 m and 125 m. Its area is
The lengths of the sides of Δ ABC are consecutive integers. It Δ ABC has the same perimeter as an equilateral triangle with a side of length 9 cm, what is the length of the shortest side of ΔABC?
The length of each side of an equilateral triangle having an area of `9sqrt(3)`cm2 is ______.
