Question

AB is a diameter of a circle and AC is its chord such that ∠BAC = 30°. If the tangent at C intersects AB extended at D, then BC = BD.

विकल्प

• True

• False

Answer 1

This statement is True.

Explanation:

Given: AB is a diameter of circle with center O and AC is a chord such that ∠BAC = 30°

Also tangent at C intersects AB extends at D.

To prove: BC = BD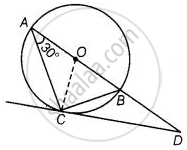

Proof: OA = OC   ...[Radii of same circle]

∠OCA = ∠OAC = 30°  ...[Angles opposite to equal sides are equal]

∠ACB = 90°   ...[Angle in a semicircle is a right angle]

∠OCA + ∠OCB = 90°

30° + ∠OCB = 90°

∠OCB = 60°  ...[1]

OC = OB   ...[Radii of same circle]

∠OBC = ∠OCB = 60° ...[Angles opposite to equal sides are equal]

Now, ∠OBC + ∠CBD = 180° ...[Linear pair]

60 + ∠CBD = 180°

So, ∠CBD = 120°  ...[2]

Also, OC ⊥ CD   ...[Tangent at a point on the circle is perpendicular to the radius through point of contact]

∠OCD = 90°

∠OCB + ∠BCD = 90°

60 + ∠BCD = 90

∠BCD = 30°  ...[3]

In ΔBCD

∠CBD + ∠BCD + ∠BDC = 180°  ...[Angle sum property of triangle]

120° + 30° + ∠BDC = 180°   ...[From 2 and 3]

∠BDC = 30°   ...[4]

From [3] and [4]

∠BCD = ∠BDC = 30°

BC = BD   ...[Sides opposite to equal angles are equal]

Hence proved.