Advertisements
Advertisements
प्रश्न
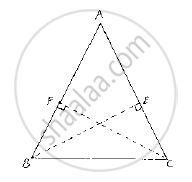
ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that ΔABC is isosceles
Advertisements
उत्तर
Given that ABC is a triangle in which BE and CF are perpendicular to the sides AC and AB respectively such that BE = CF
We have to prove that ΔABC is isosceles
Now, consider ΔBCF and ΔCBE,
We have
∠BFC=CEB=90° [Given]
BC=CB [Common side]
And CF=BE [Given]
So, by RHS congruence criterion, we have ΔBFC≅CEB
Now,
∠FBC=∠EBC [∵ Incongruent triangles corresponding parts are equal]
⇒ ∠ABC=∠ACB
⇒ AC=AB [ ∵Opposite sides to equal angles are equal in a triangle]
∴ ΔABC is isosceles
APPEARS IN
संबंधित प्रश्न
In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that:
- OB = OC
- AO bisects ∠A
In figure, AB = AC and DB = DC, find the ratio ∠ABD : ∠ACD
Determine the measure of each of the equal angles of a right-angled isosceles triangle.
AB is a line seg P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (See Fig. 10.26). Show that the line PQ is perpendicular bisector of AB.
Prove that the medians of an equilateral triangle are equal.
In a ΔABC, if ∠A=l20° and AB = AC. Find ∠B and ∠C.
If the base of an isosceles triangle is produced on both sides, prove that the exterior angles so formed are equal to each other.
ABC is a triangle and D is the mid-point of BC. The perpendiculars from D to AB and AC are equal. Prove that the triangle is isosceles.
Fill the blank in the following so that the following statement is true.
Sides opposite to equal angles of a triangle are ......
Which of the following statements are true (T) and which are false (F)?
If two angles of a triangle are unequal, then the greater angle has the larger side opposite to it.
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
In the given figure, the sides BC, CA and AB of a Δ ABC have been produced to D, E and F respectively. If ∠ACD = 105° and ∠EAF = 45°, find all the angles of the Δ ABC.
In a ΔABC, if ∠A = 60°, ∠B = 80° and the bisectors of ∠B and ∠C meet at O, then ∠BOC =
In the given figure, AB and CD are parallel lines and transversal EF intersects them at Pand Q respectively. If ∠APR = 25°, ∠RQC = 30° and ∠CQF = 65°, then
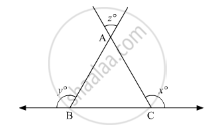
The base BC of triangle ABC is produced both ways and the measure of exterior angles formed are 94° and 126°. Then, ∠BAC =
If the bisectors of the acute angles of a right triangle meet at O, then the angle at Obetween the two bisectors is
In ∆PQR, ∠P = 70° and ∠R = 30°. Which side of this triangle is the longest? Give reason for your answer.
Find all the angles of an equilateral triangle.
Show that in a quadrilateral ABCD, AB + BC + CD + DA < 2(BD + AC)
In a triangle ABC, D is the mid-point of side AC such that BD = `1/2` AC. Show that ∠ABC is a right angle.
