Advertisements
Advertisements
प्रश्न
In the given figure, if l1 || l2, the value of x is

विकल्प
\[22\frac{1}{2}\]
30
45
60
उत्तर
In the given problem, l1 || l2
We need to find the value of x
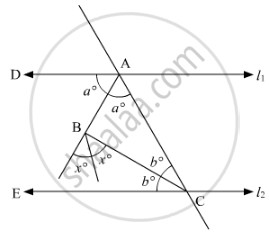
Here, as l1 || l2 , using the property, “consecutive interior angles are supplementary”, we get
∠DAC + ∠ECA = 180°
a + a+ b+ b =180°
2a + 2b = 180°
`a + b = (180°)/2`
a + b = 90° ..........(1)
Further, applying angle sum property of the triangle
In ΔABC
∠BAC + ∠BCA + ∠ABC =180°
a + b + ∠ABC = 180°
90 + ∠ABC = 180° (using 1)
∠ABC = 180° - 90°
∠ABC = 90°
Now, AB is a straight line, so using the property, “angles forming a linear pair are supplementary”, we get,
x + x + ∠ABC = 180°
2x + 90° = 180°
2x = 180° - 90°
`x = (90°)/2`
x = 45°
Thus, x = 45°
APPEARS IN
संबंधित प्रश्न
ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see the given figure). Show that these altitudes are equal.
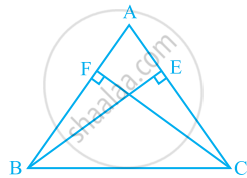
ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see the given figure). Show that
- ΔABE ≅ ΔACF
- AB = AC, i.e., ABC is an isosceles triangle.

If the base of an isosceles triangle is produced on both sides, prove that the exterior angles so formed are equal to each other.
In figure, AB = AC and DB = DC, find the ratio ∠ABD : ∠ACD
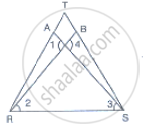
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
In a ΔABC, if ∠B = ∠C = 45°, which is the longest side?
Which of the following statements are true (T) and which are false (F)?
Difference of any two sides of a triangle is equal to the third side.
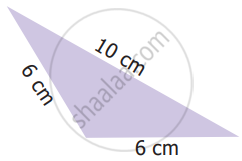
Which of the following correctly describes the given triangle?