Advertisements
Advertisements
प्रश्न
An aeroplane is flying horizontally along a straight line at a height of 3000 m from the ground at a speed of 160 m/s. Find the time it would take for the angle of elevation of the plane as seen from a particular point on the ground to change from 60⁰ to 45⁰. Give your answer correct to the nearest second.
उत्तर
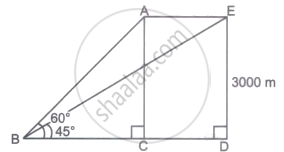
Given, AC = ED = 3,000 m
Speed = 160 m/s
In right ΔACB,
`tan 60^circ = "AC"/"BC"`
`=> sqrt3 = 3000/"BC"`
∴ BC = `3000/sqrt3`
`= 3000/sqrt3 xx sqrt3/sqrt3`
`= (3000 sqrt3)/3`
⇒ BC = `1000sqrt3` m
In right ΔEDB,
`tan 45^circ = "ED"/"BD"`
`=> 1 = 3000/"BD"`
⇒ BD = 3000 m
∴ AE = CD = BD - BC
∴ AE = 3000 - 1000`sqrt3`m
`= 1000 (3 - sqrt3)`
`= 1000 xx 1.268 ...(sqrt3 = 1.732)`
= 1268 m
∴ Time from A to E = `("Distance" ("AE"))/"Speed"`
`= 1268/160`
= 7.925 sec ≈ 8 sec
APPEARS IN
संबंधित प्रश्न
Two climbers are at points A and B on a vertical cliff face. To an observer C, 40 m from the foot of the cliff, on the level ground, A is at an elevation of 48° and B of 57°. What is the distance between the climbers?
A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60°. When he moves 40 m away from the bank, he finds the angle of elevation to be 30°. Find:
- the height of the tree, correct to 2 decimal places,
- the width of the river.
An aeroplane flying horizontally 1 km above the ground and going away from the observer is observed at an elevation of 60°. After 10 seconds, its elevation is observed to be 30°; find the uniform speed of the aeroplane in km per hour.
Find the length of the shadow cast by a tree 60 m high when the sun's altitude is `30^circ`.
A ladder rests against a tree on one side of a street. The foot of the ladder makes an angle of 50° with the ground. When the ladder is turned over to rest against another tree on the other side of the street it makes an angle of 40° with the ground. If the length of the ladder is 60m, find the width of the street.
Two boats approaching a light house in mid sea from opposite directions observe the angle of elevation of the top of the light house as 30° and 45° respectively. If the distance between the two boats is 150m, find the height of the light house.
Of the two trees are on either side of a river, one of them is 50m high. From the top of this tree the angles of depression of the top and the foot of the other tree are 30° and 60° respectively. Find the width of the river and the height of the other tree.
If the angle of elevation of a cloud from a point h m above a lake is α, and the angle of depression of its reflection in the lake be β, prove that distance of the cloud from the point of observation is `("2h"secα)/(tanα - tanβ)`.
From an aeroplane vertically above a straight horizontal road, the angles of depression of two consecutive milestone on opposite sides of the aeroplane are observed to be α, and β. Show that the height in miles of aeroplane above the road is `(tanα tanβ)/(tanα + tanβ)`.
The angle of elevation of a cloud from a point 200 metres above a lake is 30° and the angle of depression of its reflection in the lake is 60°. Find the height of the cloud.
