Advertisements
Advertisements
प्रश्न
An aeroplane is flying horizontally along a straight line at a height of 3000 m from the ground at a speed of 160 m/s. Find the time it would take for the angle of elevation of the plane as seen from a particular point on the ground to change from 60⁰ to 45⁰. Give your answer correct to the nearest second.
उत्तर
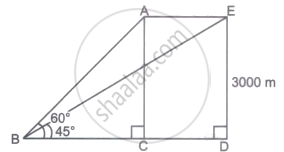
Given, AC = ED = 3,000 m
Speed = 160 m/s
In right ΔACB,
`tan 60^circ = "AC"/"BC"`
`=> sqrt3 = 3000/"BC"`
∴ BC = `3000/sqrt3`
`= 3000/sqrt3 xx sqrt3/sqrt3`
`= (3000 sqrt3)/3`
⇒ BC = `1000sqrt3` m
In right ΔEDB,
`tan 45^circ = "ED"/"BD"`
`=> 1 = 3000/"BD"`
⇒ BD = 3000 m
∴ AE = CD = BD - BC
∴ AE = 3000 - 1000`sqrt3`m
`= 1000 (3 - sqrt3)`
`= 1000 xx 1.268 ...(sqrt3 = 1.732)`
= 1268 m
∴ Time from A to E = `("Distance" ("AE"))/"Speed"`
`= 1268/160`
= 7.925 sec ≈ 8 sec
APPEARS IN
संबंधित प्रश्न
An aeroplane at an altitude of 1500 metres, finds that two ships are sailing towards it in the same direction. The angles of depression as observed from the aeroplane are 45° and 30° respectively. Find the distance between the two ships
Two vertical poles are on either side of a road. A 30 m long ladder is placed between the two poles. When the ladder rests against one pole, it makes angle 32°24′ with the pole and when it is turned to rest against another pole, it makes angle 32°24′ with the road. Calculate the width of the road.
From the top of a light house 100 m high, the angles of depression of two ships are observed as 48° and 36° respectively. Find the distance between the two ships (in the nearest metre) if:
- the ships are on the same side of the light house,
- the ships are on the opposite sides of the light house.
A man standing on the bank of a river observes that the angle of elevation of a tree on the opposite bank is 60°. When he moves 50 m away from the bank, he finds the angle of elevation to be 30°.
Calculate :
- the width of the river;
- the height of the tree.
The length of the shadow of a pillar is `1/sqrt(3)` times the height of the pillar . find the angle of elevation of the sun .
A kite tied to a string makes an angle of 60° with the ground. Find the perpendicular height of the kite if the length of its string is 250 m.
The angles of depression of two cars on a straight road as observed from the top of a 42m high building are 60° and 75° respectively. Find the distance between the cars if they are on opposite sides of the building.
The angle of elevation of a tower from a point in line with its base is `45^circ` . On moving 20m towards the tower , the angle of elevation changes to `60^circ` . Find the height of the tower.
A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole, the angle of elevation of the top of the tower is 60o and the angle of depression of the bottom of the tower is 30o. Find: the height of the pole, if the height of the tower is 75 m.
From the top of a lighthouse 100 m high the angles of depression of two ships on opposite sides of it are 48° and 36° respectively. Find the distance between the two ships to the nearest metre.

