Advertisements
Advertisements
प्रश्न
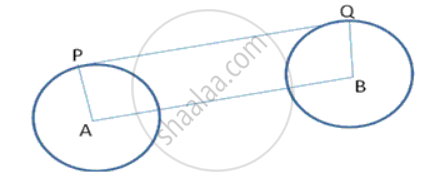
Calculate the length of direct common tangent to two circles of radii 3cm and Bern with their centres 13cm apart.
उत्तर
To find: PQ
R1 = 3cm, R2 = 8cm
AB= 13cm
PQ2 = AB2 -(R2 -R1)2
⇒ PQ2 = 132 - ( 8 - 3)2
⇒ PQ2 = 169 - 25
⇒ PQ2 = 144
⇒ PQ = 12cm
Length of direct common tangent is 12cm
APPEARS IN
संबंधित प्रश्न
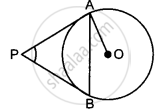
If the angle between two tangents drawn from an external point P to a circle of radius a and centre O, is 60°, then find the length of OP
In Figure 3, a right triangle ABC, circumscribes a circle of radius r. If AB and BC are of lengths of 8 cm and 6 cm respectively, find the value of r.

Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.
Find the length of the tangent from a point which is at a distance of 5cm from the centre of the circle of radius 3cm.
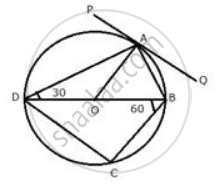
In the following figure, PQ is the tangent to the circle at A, DB is a diameter and O is the centre of the circle. If ∠ ADB = 30° and ∠ CBD = 60° ; calculate ∠ PAD.
In the following figure, seg AB is a diameter of the circle, m (arc AKC) = 40°. Find the value of m (arc BMC).

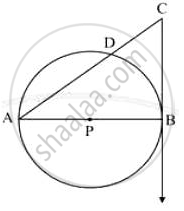
In the following figure, seg AB is the diameter of the circle with center P. Line CB be the tangent and line AC intersects a circle in point D. Prove that:
AC x AD = 4 (radius)2
In Fig. the incircle of ΔABC touches the sides BC, CA, and AB at D, E respectively. Show that: AF + BD + CE = AE + BF + CD = `1/2`( Perimeter of ΔABC)

In the figure PA and PB are tangents to the circle with centre O. If ∠APB = 60°, then ∠OAB is ______
In the given figure, PT is a tangent to the circle at T, chord BA is produced to meet the tangent at P. Perpendicular BC bisects the chord TA at C. If PA = 9 cm and TB = 7 cm, find the lengths of:
- AB
- PT

