Advertisements
Advertisements
प्रश्न
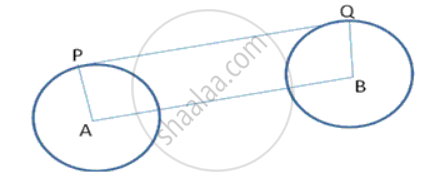
Calculate the length of direct common tangent to two circles of radii 3cm and Bern with their centres 13cm apart.
उत्तर
To find: PQ
R1 = 3cm, R2 = 8cm
AB= 13cm
PQ2 = AB2 -(R2 -R1)2
⇒ PQ2 = 132 - ( 8 - 3)2
⇒ PQ2 = 169 - 25
⇒ PQ2 = 144
⇒ PQ = 12cm
Length of direct common tangent is 12cm
APPEARS IN
संबंधित प्रश्न
A circle touches all the four sides of a quadrilateral ABCD. Prove that AB + CD = BC + DA.
In Fig. 1, AOB is a diameter of a circle with centre O and AC is a tangent to the circle at A. If ∠BOC = 130°, the find ∠ACO.

A chord of a length 16.8 cm is at a distance of 11.2 cm from the centre of a circle . Find the length of the chord of the same circle which is at a distance of 8.4 cm from the centre.
Two chords AB and CD of lengths 6cm and 12cm are drawn parallel inside the circle. If the distance between the chords of the circle is 3cm, find the radius of the circle.
Find the length of the tangent from a point which is at a distance of 5cm from the centre of the circle of radius 3cm.
In the alongside, figure, O is the centre of the circumcircle of triangle XYZ. Tangents at X and Y intersect at T. Given ∠XTY = 80° and ∠XOZ = 140°. Calculate the value of ∠ZXY.

The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
In the adjoining figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 30°, find the value of x.

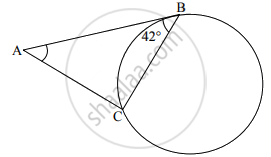
In the given figure, AB and AC are tangents to the circle. If ∠ABC = 42°, then the measure of ∠BAC is ______.
In the adjoining diagram, O is the centre of the circle and PT is a tangent. The value of x is ______.

