Advertisements
Advertisements
प्रश्न
In the adjoining figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 30°, find the value of x.

उत्तर
Given that,
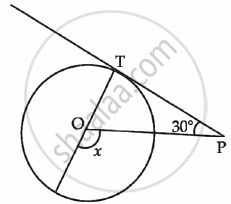
PT is a tangent at T to circle
Also, ∠TPO = 30°
So, TPO is right-angled triangle with ∠T = 90°
We have, ∠POT = (180°) – (30° + 90°) = 60°
As, x + ∠POT = 180° (linear pair angles)
`\implies` x = 180° – 120° = 60°
APPEARS IN
संबंधित प्रश्न
A chord PQ of a circle is parallel to the tangent drawn at a point R of the circle. Prove that R bisects the arc PRQ.
The length of the direct common tangent to two circles of radii 12cm and 4cm is 15cm. calculate the distance between their centres.
Construct a tangent to a circle with centre O and radius 3.5 cm at a point P on it.
In the following figure, seg AB is a diameter of the circle, m (arc AKC) = 40°. Find the value of m (arc BMC).

If PA and PB are two tangents drawn from a point P to a circle with center C touching it A and B, prove that CP is the perpendicular bisector of AB.
In the figure given below, PT is a tangent to the circle. Find PT if AT = 16 cm and AB = 12 cm.

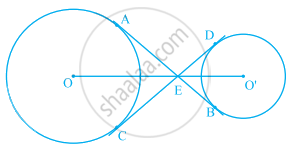
In figure, the common tangent, AB and CD to two circles with centres O and O' intersect at E. Prove that the points O, E, O' are collinear.
A tangent JK is drawn to a circle with centre C such that CK = 6 cm and ∠CKJ = 60°. Find the length of the tangent JK.

Construct a pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 60°.
ΔABC circumscribes a circle of radius r such that ∠B = 90°. If AB = 3 cm and BC = 4 cm, then find the value of r.
