Advertisements
Advertisements
प्रश्न
Figure shown a two slit arrangement with a source which emits unpolarised light. P is a polariser with axis whose direction is not given. If I0 is the intensity of the principal maxima when no polariser is present, calculate in the present case, the intensity of the principal maxima as well as of the first minima.
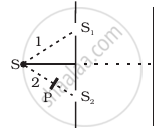
उत्तर
The resultant amplitude of wave reaching the screen will be the sum of amplitude of either wave in perpendicular and parallel polarisation.
Amplitude of the wave in perpendicular polarisation
`A_⊥ = A_⊥^1 + A_⊥^2 = A_⊥^0 sin (kx - ωt) + A_⊥^0 sin (kx - ωt + phi)`
⇒ `A_⊥ = A_⊥^0 (sin (kx - ωt) + sin (kx - ωt + phi)`
Amplitude of the wave in parallel polarisation
`A_(||) = A_(||)^((1)) + A_(||)^((2))`
⇒ `A_(||) = A_(||)^0 [sin (kx - ωt) + sin (kx - ωt + phi)]`
∴ Average Intensity on the screen
`I = {|A_⊥^0|^2 + |A_(||)^0|^2} [sin^2 (kx - ωt)(1 + cos^2 phi + 2 sin phi) + sin^2 (kx - ωt) sin^2 phi]_("average")`
= `{|A_⊥^0|^2 + |A_(||)^0|^2}(1/2)*2(1 + cos phi)`
⇒ `I = 2|A_⊥^0|^2 (1 + cos phi)` since, `|A_⊥^0|_("av") = |A_(||)^0|_("av")`
With polariser P,
Assume `A_⊥^2` is blocked
Intensity = `(A_(||)^1 + A_(||)^2)^2 + (A_⊥^1)^2`
= `|A_⊥^2|^2 (1 + cos phi) + |A_⊥^0|^2 * 1/2`
Given, `I_0 4|A_⊥^0|^2` = Intensity without polariser at principal maxima.
Intensity at first minima with polariser = `|A_⊥^0|^2 (1 - 1) + (|A_⊥^0|^2)/2 = I_0/8`.
APPEARS IN
संबंधित प्रश्न
For a glass plate as a polariser with refractive index 1.633, calculate the angle of incidence at which light is polarised.
A ray of light passes from a vacuum to a medium of refractive index (μ). The angle of
incidence is found to be twice the angle of refraction. The angle of incidence is _______.
A) `cos^(-1)(mu/2)`
B) cos−1(μ)
C) `2 cos^(-1) (mu/2)`
D) `2 sin^(-1) (mu/2)`
With the help of an experiment, state how will you identify whether a given beam of light is polarised or unpolarized?
A ray of light is incident on a transparent medium at a polarizing angle. What is the angle between the reflected ray and the refracted ray?
What is plane polarised light?
State and obtain Malus’ law.
What is the angle of polarisation and obtain the equation for an angle of polarisation?
Mention the types of optically active crystals with example.
Discuss about Nicol prism.
The reflected light is found to be plane polarised when an unpolarized light falls on a denser medium at 60° with the normal. Find the angle of refraction and critical angle of incidence for total internal reflection in the denser to rarer medium reflection.
