Advertisements
Advertisements
प्रश्न
Find the length of the chord of a circle in the following when:
Radius is 1. 7cm and the distance from the centre is 1.5 cm
उत्तर
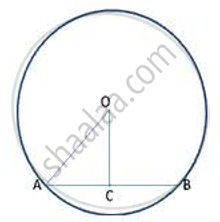
AC = CB ----(1) (Perpendicular from centre to a chord bisects the chord)
In right Δ ACO,
By Pythagoras theorem, OA2 = OC2 + AC2
AC2 = (1. 7)2 - (1.5)2 = 2.89 - 2.25 =.64
AC = 0.8 cm
∴ length of chord AB = 2AC (from ( 1))
= 2(0.8) = 1.6 cm
APPEARS IN
संबंधित प्रश्न
In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP

Fill in the blanks:
A circle divides the plane, on which it lies, in __________ parts.
true or false
Sector is the region between the chord and its corresponding arc.
In Fig. 4, an isosceles triangle ABC, with AB = AC, circumscribes a circle. Prove that the point of contact P bisects the base BC.
Construct a triangle PQR in which, PQ = QR = RP = 5.7 cm. Draw the incircle of the triangle and measure its radius.
A line segment joining any point on the circle to its center is called the _____________ of the circle
In a circle with centre P, chord AB is parallel to a tangent and intersects the radius drawn from the point of contact to its midpoint. If AB = `16sqrt(3)`, then find the radius of the circle
If a number of circles pass through the endpoints P and Q of a line segment PQ, then their centres lie on the perpendicular bisector of PQ.
The tangent at a point C of a circle and a diameter AB when extended intersect at P. If ∠PCA = 110°, find ∠CBA see figure
If ABC is an equilateral triangle inscribed in a circle and P be any point on the minor arc BC which does not coincide with B or C, prove that PA is angle bisector of ∠BPC.
