Advertisements
Advertisements
प्रश्न
Find the length of the support cable required to support the tower with the floor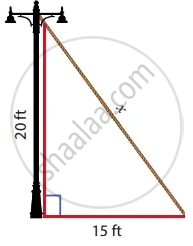
उत्तर
From the figure, by Pythagoras theorem,
x2 = 202 + 152
= 400 + 225
= 625
x2 = 252
⇒ x = 25 feet.
∴ The length of the support cable required to support the tower with the floor is 25 feet.
APPEARS IN
संबंधित प्रश्न
If ABC is an equilateral triangle of side a, prove that its altitude = ` \frac { \sqrt { 3 } }{ 2 } a`
Walls of two buildings on either side of a street are parallel to each other. A ladder 5.8 m long is placed on the street such that its top just reaches the window of a building at the height of 4 m. On turning the ladder over to the other side of the street, its top touches the window of the other building at a height 4.2 m. Find the width of the street.
Some question and their alternative answer are given. Select the correct alternative.
If a, b, and c are sides of a triangle and a2 + b2 = c2, name the type of triangle.
In an isosceles triangle, length of the congruent sides is 13 cm and its base is 10 cm. Find the distance between the vertex opposite the base and the centroid.
In the given figure, ∠B = 90°, XY || BC, AB = 12 cm, AY = 8cm and AX : XB = 1 : 2 = AY : YC.
Find the lengths of AC and BC.

In triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC.

In triangle ABC, angle A = 90o, CA = AB and D is the point on AB produced.
Prove that DC2 - BD2 = 2AB.AD.
In triangle ABC, ∠B = 90o and D is the mid-point of BC.
Prove that: AC2 = AD2 + 3CD2.
Prove that in a right angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
In an equilateral triangle PQR, prove that PS2 = 3(QS)2.

