Topics
Number Systems
Real Numbers
Algebra
Polynomials
Pair of Linear Equations in Two Variables
- Introduction to linear equations in two variables
- Graphical Method
- Substitution Method
- Elimination Method
- Cross - Multiplication Method
- Equations Reducible to a Pair of Linear Equations in Two Variables
- Consistency of Pair of Linear Equations
- Inconsistency of Pair of Linear Equations
- Algebraic Conditions for Number of Solutions
- Simple Situational Problems
- Pair of Linear Equations in Two Variables
- Relation Between Co-efficient
Quadratic Equations
- Quadratic Equations
- Solutions of Quadratic Equations by Factorization
- Solutions of Quadratic Equations by Completing the Square
- Nature of Roots of a Quadratic Equation
- Relationship Between Discriminant and Nature of Roots
- Situational Problems Based on Quadratic Equations Related to Day to Day Activities to Be Incorporated
- Application of Quadratic Equation
Arithmetic Progressions
Coordinate Geometry
Lines (In Two-dimensions)
Constructions
- Division of a Line Segment
- Construction of Tangents to a Circle
- Constructions Examples and Solutions
Geometry
Triangles
- Similar Figures
- Similarity of Triangles
- Basic Proportionality Theorem (Thales Theorem)
- Criteria for Similarity of Triangles
- Areas of Similar Triangles
- Right-angled Triangles and Pythagoras Property
- Similarity of Triangles
- Application of Pythagoras Theorem in Acute Angle and Obtuse Angle
- Triangles Examples and Solutions
- Concept of Angle Bisector
- Similarity of Triangles
- Ratio of Sides of Triangle
Circles
Trigonometry
Introduction to Trigonometry
- Trigonometry
- Trigonometry
- Trigonometric Ratios
- Trigonometric Ratios and Its Reciprocal
- Trigonometric Ratios of Some Special Angles
- Trigonometric Ratios of Complementary Angles
- Trigonometric Identities
- Proof of Existence
- Relationships Between the Ratios
Trigonometric Identities
Some Applications of Trigonometry
Mensuration
Areas Related to Circles
- Perimeter and Area of a Circle - A Review
- Areas of Sector and Segment of a Circle
- Areas of Combinations of Plane Figures
- Circumference of a Circle
- Area of Circle
Surface Areas and Volumes
- Surface Area of a Combination of Solids
- Volume of a Combination of Solids
- Conversion of Solid from One Shape to Another
- Frustum of a Cone
- Concept of Surface Area, Volume, and Capacity
- Surface Area and Volume of Different Combination of Solid Figures
- Surface Area and Volume of Three Dimensional Figures
Statistics and Probability
Statistics
Probability
Internal Assessment
Formula
Pythagoras Theorem:
(Hypotenuse)2 = (base)2 + (height)2
Notes
1. Right-Angled Triangles:
- Pythagoras, a Greek philosopher of the sixth century B.C. is said to have found a very important and useful property of right-angled triangles. The property is, hence, named after him.
- The Indian mathematician Baudhayan has also given an equivalent form of this property.
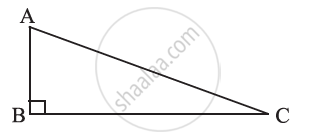
- The side opposite to the right angle is called the hypotenuse; the other two sides are known as the legs of the right-angled triangle. In any right-angled triangle, the hypotenuse happens to be the longest side.
- In ∆ABC, the right-angle is at B. So, AC is the hypotenuse. `bar"AB" and bar"BC"` are the legs of ∆ABC.
2. Pythagoras Property:
(i) In a right-angled triangle, the square on the hypotenuse = sum of the squares on the legs.
From a card sheet, cut out eight identical right-angled triangles. Let us say the length of the hypotenuse of these triangles is ‘a’ units, and sides forming the right angle are ‘b’ and ‘c’ units. Note that the area of this triangle is `"bc"/2`. Next, on another card sheet, use a pencil to draw two squares ABCD and PQRS each of side (b + c) units. Now, place 4 of the triangle cut-outs in the square ABCD and the remaining 4 in the square PQRS as shown in the figures below. Mark by lines drawn across them, the parts of the squares covered by the triangles.
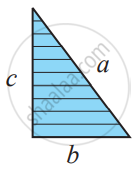

Observe the figures. In figure (i) we can see a square of side a units in the uncovered portion of square ABCD. In figure (ii) we see a square of side b and another of side c in the uncovered portion of the square PQRS.
In figure (i), area of square ABCD = a2 + 4 × area of right-angled triangle
= a2 + 4 x `1/2`bc
= a2 + 2bc
In figure (ii), area of square PQRS = b2 + c2 + 4 × area of right-angled triangle
= b2 + c2 + 4 × `1/2` bc
= b2 + c2 + 2bc
Area of square ABCD = Area of square PQRS
∴ a2 + 2bc = b2 + c2 + 2bc
∴ a2 = b2 + c2
(ii) Any right-angled triangle, the area of the square on the hypotenuse is equal to the sum of the areas of the squares on the legs.
Draw a right triangle, preferably on a square sheet, construct squares on its sides, compute the area of these square.
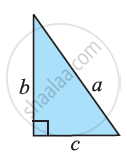
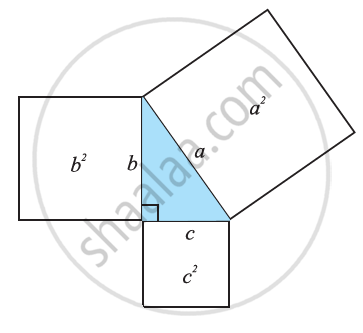
a2 =b2 + c2
We will show that, if there is a triangle such that the sum of the squares on two of its sides is equal to the square of the third side, it must be a right-angled triangle.
If the Pythagoras property holds, the triangle must be right-angled.
Pythagoras property says that for any right-angled triangle, the area of the square on the hypotenuse is equal to the sum of the areas of the squares on the legs.
Example
Determine whether the triangle whose lengths of sides are 3 cm, 4 cm, 5 cm is a right-angled triangle.
32 = 3 × 3 = 9;
42 = 4 × 4 = 16;
52 = 5 × 5 = 25
We find 32 + 42 = 52.
Therefore, the triangle is right-angled.
Example
∆ABC is right-angled at C. If AC = 5 cm and BC = 12 cm. find the length of AB.
A rough figure will help us.
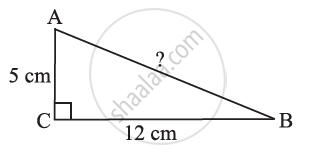
By Pythagoras property,
AB2 = AC2 + BC2
AB2 = 52 + 122
AB2 = 25 + 144
AB2 = 169
AB2 = 132
AB2 =13.
So, AB = 13 or the length of AB is 13 cm.
Video Tutorials
Shaalaa.com | Triangles part 39 (Proof Converse of Pythagoras Theorem)
Series: 1
00:03:31 undefined
00:06:49 undefined
00:08:00 undefined
00:11:12 undefined
00:11:47 undefined
00:09:18 undefined
00:11:34 undefined
00:12:03 undefined



