Advertisements
Advertisements
प्रश्न
Find the volume of the largest right circular cone that can be cut out of a cube where edgeis 9cm?
उत्तर
We have the following figure
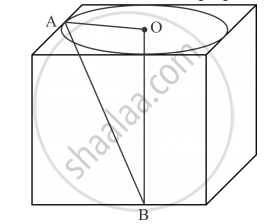
The length of each side of the cube is 9 cm. We have to find the volume of the largest right circular cone contained in the cube.
The diameter of the base circle is same as the length of the side of the cube. Thus, the diameter of the base circle of the right circular cone is 9 cm. Therefore, the radius of the base of the right circular cone is r = 4.5cm.
From the right angled triangle ΔAOB we have
h = 9 cm
Therefore, the volume of the solid right circular cone is
`V = 1/3 pir^2h`
`= 1/3 xx 22/7 xx (4.5)^2 xx 9`
` = 190.93`
Hence largest volume of cone is ` = 190.93cm^3`
APPEARS IN
संबंधित प्रश्न
A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm3 of iron has approximately 8 g mass. [Use π = 3.14]
A spherical glass vessel has a cylindrical neck 8 cm long, 2cm in diameter; the diameter of the spherical part is 8.5 cm. By measuring the amount of water it holds, a child finds its volume to be 345 cm3. Check whether she is correct, taking the above as the inside measurements, and π = 3.14
The radii of two cylinders are in the ratio 3 : 5. If their heights are in the ratio 2 : 3, then the ratio of their curved surface areas is
From a solid cylinder of height 14 cm and base diameter 7 cm, two equal conical holes each of radius 2.1 cm and height 4 cm are cut off. Find the volume of the remaining solid.
150 spherical marbles, each of diameter 1.4 cm, are dropped in a cylindrical vessel of diameter 7 cm containing some water, which are completely immersed in water. Find the rise in the level of water in the vessel.
The given figure shows a solid formed of a solid cube of side 40 cm and a solid cylinder of radius 20 cm and height 50 cm attached to the cube as shown.

Find the volume and the total surface area of the whole solid. [Take π = 3.14].
In the equilateral Δ ABC of side 14 cm, side BC is the diameter of a semicircle as shown in the figure below. Find the area of the shaded region. (Take π = 22/7 and √3 = 1.732)
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
A student was asked to make a model shaped like a cylinder with two cones attached to its ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its total length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model.
From a soild cylinder of height 20 cm and diameter 12 cm, a conical cavity of height 8 cm and radius 6 cm is hallowed out. Find the total surface area of the remaining solid.
