Advertisements
Advertisements
प्रश्न
If cos A = `(1)/(2)` and sin B = `(1)/(sqrt2)`, find the value of: `(tan"A" – tan"B")/(1+tan"A" tan"B")`.
Are angles A and B from the same triangle? Explain.
उत्तर
Consider the diagram below:
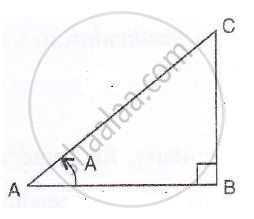
cos A = `(1)/(2)`
i.e.`"base"/"hypotenuse"= (1)/(2)`
⇒ `"AB"/"AC" = (1)/(2)`
Therefore if length of AB = x, length of AC = 2x
Since
AB2 + BC2 = AC2 ...[Using Pythagoras Theorem]
(x)2 + BC2 = (2x)2
BC2 = 4x2 – x2 = 3x2
∴ BC = `sqrt3x` ...(perpendicular)
Consider the diagram below:

sin B = `(1)/(sqrt2)`
i.e.`"perpendicular"/"hypotenuse" = (1)/(sqrt2)`
⇒ `"AC"/"BC" = (1)/(sqrt2)`
Therefore if length of AC = x, length of BC = `sqrt2`
Since
AB2 + AC2 = BC2 ...[Using Pythagoras Theorem]
AB2 + x2 = `(sqrt2x)^2`
AB2 = 2x2 – x2 = x2
∴ AB = x (base)
Now
tan A = `"perpendicular"/"base" = (sqrt3x)/(x) = sqrt3`
tan B =`"perpendicular"/"base" = (x)/(x) =1`
Thererfore
`(tan"A" – tan"B")/(1+tan"A"tan"B")`
= `(sqrt3 – 1)/(1+sqrt3)`
= `(sqrt3 – 1)/(1+sqrt3) xx (1-sqrt3) /(1-sqrt3)`
= `((sqrt3 - 1) (1-sqrt3))/(1-3) `
= `(sqrt3 - 3 - 1 + sqrt3) / 2`
= `(2sqrt3-4)/2`
= `(cancel2 (sqrt3-2))/cancel2`
= `sqrt3 -2`
APPEARS IN
संबंधित प्रश्न
If `sin (A – B) = 1/2` and `cos (A + B) = 1/2`, `0^@` < A + `B <= 90^@`, A > B Find A and B.
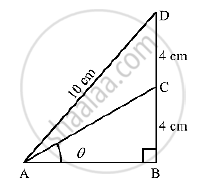
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
Evaluate:
`(sin^2 30^0 + 4 cot^2 45^0-sec^2 60^0)(cosec^2 45^0 sec^2 30^0)`
Show that:
(i)` (1-sin 60^0)/(cos 60^0)=(tan60^0-1)/(tan60^0+1)`
If A = 600 and B = 300, verify that:
(iii) tan (A-B) = `(tan A-tanB)/(1+tan A tan B)`
If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
If 5 cos = 6 sin ; evaluate:
(i) tan θ
(ii) `(12 sin θ – 3 cos θ)/(12 sin θ + 3 cos θ)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cos A = `(7)/(25)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cosec C = `sqrt(10)`
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of `(1)/("sin"^2 x) - (1)/("tan"^2 x)`
