Advertisements
Advertisements
प्रश्न
Given: sin θ = `p/q`.
Find cos θ + sin θ in terms of p and q.
उत्तर
Consider the diagram below :

sin θ = `p/q`
i.e.`"perpendicular"/"hypotenuse" = p/h`
Therefore if length of perpendicular = px,
length of hypotenuse = qx
Since
hypotenuse2 = base2 + perpendicular2 ...[Using Pythagoras Theorem]
(qx)2 = base2 + (px)2
q2x2 = p2x2 + base2
q2x2 - p2x2 = base2
(q2 – p2)x2 = base2
∴ base = `sqrt(("q"^2 – "p"^"2")x^2)`
∴ base = `xsqrt("q"^2 - "p"^2) = "base"`
Now
cos θ = `"base"/"hypotenuse" = (xsqrt(q^2 – p^2))/(qx)`
Therefore, cosθ + sinθ
= `(xsqrt(q^2 – p^2))/(qx) + p/q`
= `(sqrt(q^2 – p^2))/(q) + p/q`
= `(p + sqrt(q^2 – p^2))/q`
APPEARS IN
संबंधित प्रश्न
if `sec theta = 5/4` find the value of `(sin theta - 2 cos theta)/(tan theta - cot theta)`
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
If sin θ ,` sqrt (3)/2` find the value of all T- ratios of θ .
If cosec θ = `sqrt(10)` find all the values of all T-ratios of θ
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

If A = 300 , verify that:
(ii) cos 2A = `(1- tan^2A)/(1+tan^2A)`
tan 30° × tan ______° = 1
In the diagram, given below, triangle ABC is right-angled at B and BD is perpendicular to AC.
Find:
(i) cos ∠DBC
(ii) cot ∠DBA

From the given figure, find the values of tan C
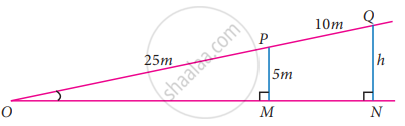
A boy standing at a point O finds his kite flying at a point P with distance OP = 25 m. It is at a height of 5 m from the ground. When the thread is extended by 10 m from P, it reaches a point Q. What will be the height QN of the kite from the ground? (use trigonometric ratios)