Advertisements
Advertisements
प्रश्न
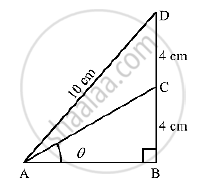
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
उत्तर

In ΔABD,
Using Pythagoras theorem, we get
AB= `sqrt(AD^2-BD^2)`
= `sqrt(10^2-8^2)`
=`sqrt(100-64)`
=`sqrt(36)`
=6cm
Again,
In ΔABC,
Using Pythagoras therem, we get
AC= `sqrt(AB^2 +BC^2)`
=`sqrt(6^2+4^2)`
=`sqrt(36+16)`
=`sqrt(52)`
=2`sqrt(13)`cm
Now,
(i) `sintheta = (BC)/(AC)`
=`4/(2sqrt(13))`
=`2/sqrt(13)`
=`(2 sqrt(13))/13`
(ii) `cos theta = (AB)/(AC)`
= `6/(2sqrt(13))`
=`3/sqrt(13)`
=`(3sqrt(13))/13`
APPEARS IN
संबंधित प्रश्न
If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P.
If A = 30° and B = 60°, verify that cos (A + B) = cos A cos B − sin A sin B
If sin θ = `a/b`, show that `(sectheta + tan theta) = sqrt((b+a)/(b-a))`
In the adjoining figure, ΔABC is a right-angled triangle in which ∠B = 900, ∠300 and AC = 20cm. Find (i) BC, (ii) AB.
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
If cos A = `(1)/(2)` and sin B = `(1)/(sqrt2)`, find the value of: `(tan"A" – tan"B")/(1+tan"A" tan"B")`.
Are angles A and B from the same triangle? Explain.
In the given figure; ∠C = 90o and D is mid-point of AC.
Find :
(i) `(tan∠CAB)/ (tan∠CDB)` (ii) `(tan∠ABC)/ (tan∠DBC)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cose C = `(15)/(11)`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`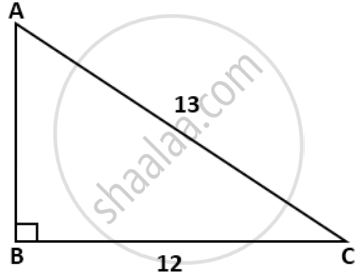
Evaluate: `5/(cot^2 30^circ) + 1/(sin^2 60^circ) - cot^2 45^circ + 2 sin^2 90^circ`.
