Advertisements
Advertisements
प्रश्न
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`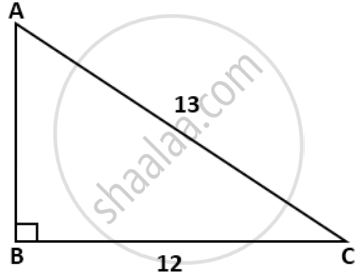
उत्तर
ΔABC is a right-angled triangle.
∴ AC2 = AB2 + BC2
⇒ AB2
= AC2 - BC2
= 132 - 122
= 169 - 144
= 25
⇒ AB = 5cm
sin A = `"BC"/"AC" = (12)/(13)`
cos A = `"AB"/"AC" = (5)/(13)`
`("cos A" - "sin A")/("cos A" + "sin A")`
= `(5/13 - 12/13)/(5/13 + 12/13)`
= `(-(7)/(13))/((17)/(13)`
= `-(7)/(13) xx (13)/(17)`
= `-(7)/(17)`.
APPEARS IN
संबंधित प्रश्न
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
If tan θ =`15/ 8 `, find the values of all T-ratios of θ.
If cot θ = 2 find all the values of all T-ratios of θ .
If ∠A and ∠B are acute angles such that tan A= Tan B then prove that ∠A = ∠B
In the figure of ΔPQR , ∠P = θ° and ∠R =∅° find
(i) `sqrt(X +1) cot ∅`
(ii)`sqrt( x^3 + x ^2) tantheta`
(iii) cos θ

If sin (A – B) = `1/2` and cos (A + B) = `1/2, 0^0 ≤ (A + B) ≤ 90^0` and A > B, then find A and B.
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: tan A
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cos C
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: cot C
If sinA = `(3)/(5)`, find cosA and tanA.
