Advertisements
Advertisements
Question
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`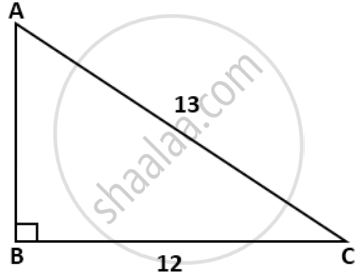
Solution
ΔABC is a right-angled triangle.
∴ AC2 = AB2 + BC2
⇒ AB2
= AC2 - BC2
= 132 - 122
= 169 - 144
= 25
⇒ AB = 5cm
sin A = `"BC"/"AC" = (12)/(13)`
cos A = `"AB"/"AC" = (5)/(13)`
`("cos A" - "sin A")/("cos A" + "sin A")`
= `(5/13 - 12/13)/(5/13 + 12/13)`
= `(-(7)/(13))/((17)/(13)`
= `-(7)/(13) xx (13)/(17)`
= `-(7)/(17)`.
APPEARS IN
RELATED QUESTIONS
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
If sin ∝ = `1/2` prove that (3cos∝ - `4cos^2` ∝)=0
If x = cosec A +cos A and y = cosec A – cos A then prove that `(2/(x+y))^2 + ((x-y)/2)^2` = 1
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
From the following figure, find the values of:
- sin A
- cos A
- cot A
- sec C
- cosec C
- tan C

In the diagram, given below, triangle ABC is right-angled at B and BD is perpendicular to AC.
Find:
(i) cos ∠DBC
(ii) cot ∠DBA

If cosec θ = `(29)/(20)`, find the value of: cosec θ - `(1)/("cot" θ)`
Statement A (Assertion): For 0 < θ ≤ 90°, cosec θ – cot θ and cosec θ + cot θ are reciprocal of each other.
Statement R (Reason): cosec2 θ – cot2 θ = 1
