Advertisements
Advertisements
प्रश्न
If sinA = `(3)/(5)`, find cosA and tanA.
उत्तर
sinA = `(3)/(5) = "Perpendicular"/"Hypotenuse"`
By Pythagoras theorem, we have
⇒ (Hypotenuse)2 = (Perpendicular)2 + (Base)2
⇒ (Base)2 = (Hypotenuse)2 - (Perpendicular)2
⇒ (Base) = `sqrt(("Hypotenuse")^2 - ("Perpendicular")^2`
⇒ (Base)
= `sqrt(5^2 - 3^2)`
= `sqrt(25 - 9)`
= `sqrt(16)`
= 4
cos A = `"Base"/"Hypotenuse" = (4)/(5)`
tan A = `"Perpendicular"/"Base" = (3)/(4)`.
APPEARS IN
संबंधित प्रश्न
If A = B = 60°. Verify `tan (A - B) = (tan A - tan B)/(1 + tan tan B)`
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
If θ is a positive acute angle such that sec θ = cosec 60°, find 2 cos2 θ – 1
If cosec θ = `sqrt(10)` find all the values of all T-ratios of θ
If tan θ = `20/21` show that `((1-sin θ + cos θ))/((1+ sin θ +cos θ)) = 3/7`
If cos A = `(1)/(2)` and sin B = `(1)/(sqrt2)`, find the value of: `(tan"A" – tan"B")/(1+tan"A" tan"B")`.
Are angles A and B from the same triangle? Explain.
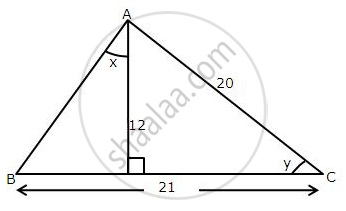
Use the information given in the following figure to evaluate:
`(10)/sin x + (6)/sin y – 6 cot y`.
If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: sin P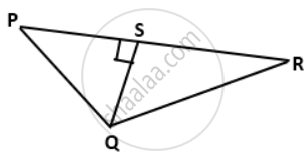
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`