Advertisements
Advertisements
प्रश्न
If sin θ = `a/b`, show that `(sectheta + tan theta) = sqrt((b+a)/(b-a))`
उत्तर
Given: `sintheta = a/b`
To show: `sec thetatantheta = sqrt((b + a)/(b - a))`
Using Trigonometric identity,
`costheta = sqrt(1 - sin^2 theta)`
`costheta = sqrt(1 - (a/b)^2)`
`costheta = sqrt((b^2 - a^2)/(b^2))`
`costheta = sqrt((b^2 - a^2)/(b))`
⇒ `sectheta = 1/(costheta) = b/(sqrt(b^2 - a^2))`
⇒ `tan theta = (sintheta)/(costheta) = a/(sqrt(b^2 - a^2))`
Now,
`sec theta + tan theta`
= `b/(sqrt(b^2 - a^2)) + a/(sqrt(b^2 - a^2))`
= `(b + a)/(sqrt((b - a)(b + a)))`
= `(sqrt(b + a)sqrt(b + a))/(sqrt(b - a)sqrt(b + a))`
= `(sqrt(b + a))/(sqrt(b - a))`
= `sqrt((b + a)/(b - a))`
APPEARS IN
संबंधित प्रश्न
In Fig below, Find tan P and cot R. Is tan P = cot R?

If 3cos θ – 4sin = 2cos θ + sin θ Find tan θ.
In a ΔABC, right angled at A, if tan C = `sqrt3` , find the value of sin B cos C + cos B sin C.
If sin θ ,` sqrt (3)/2` find the value of all T- ratios of θ .
Given: cos A = `( 5 )/ ( 13 )`
Evaluate:
- `(sin "A "–cot "A") / (2 tan "A")`
- `cot "A" + 1/cos"A"`
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
If sin A = cos A, find the value of 2 tan2A - 2 sec2 A + 5.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cos A = `(7)/(25)`
From the given figure, find the values of sec B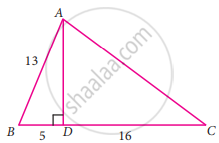
If 3 cot A = 2, then find the value of `(4sin"A" - 3cos"A")/(2sin"A" + 3cos"A")`
