Advertisements
Advertisements
प्रश्न
If 3 cot A = 2, then find the value of `(4sin"A" - 3cos"A")/(2sin"A" + 3cos"A")`
उत्तर
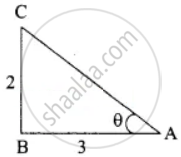
3 cot A = 2
⇒ cot A = `2/3`
AC2 = AB2 + BC2
= 32 + 22
= 9 + 4
AC = `sqrt(13)`
cos A = `"AB"/"AC" = 3/sqrt(13)`
sin A = `"BC"/"AC" = 2/sqrt(13)`
The value of `(4sin"A" - 3cos"A")/(2sin"A" + 3cos"A")`
= `4(2/sqrt(13)) - 3(3/sqrt(13)) ÷ 2(2/sqrt(13)) + 3(3/sqrt(13))`
= `(8/sqrt(13)) - (9/sqrt(13)) ÷ (4/sqrt(13)) + (9/sqrt(13))`
= `((8 - 9)/sqrt(13)) ÷((4 + 9)/sqrt(13))`
= `((-1)/sqrt(13)) ÷ (3/sqrt(13))`
= `((-1)/sqrt(13)) xx (sqrt(13)/13)`
The value is `((-1)/13)`
APPEARS IN
संबंधित प्रश्न
if `sec theta = 5/4` find the value of `(sin theta - 2 cos theta)/(tan theta - cot theta)`
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
If cot θ = 2 find all the values of all T-ratios of θ .
If sin A = `9/41` find all the values of cos A and tan A
If cot θ = `3/4` , show that `sqrt("sec θ - cosecθ"/"secθ + cosecθ" ) = 1/ sqrt(7)`
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
Evaluate:
`cot^2 30^0-2cos^2 30^0-3/4 sec^2 45^0 +1/4 cosec^2 30^0`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sec B = `(15)/(12)`
A boy standing at a point O finds his kite flying at a point P with distance OP = 25 m. It is at a height of 5 m from the ground. When the thread is extended by 10 m from P, it reaches a point Q. What will be the height QN of the kite from the ground? (use trigonometric ratios)