Advertisements
Advertisements
प्रश्न
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
उत्तर
We know that sin (90 - θ) = cos θ
sin 20 = cos 2θ
sin 4θ = sin (90 - 2θ)
4θ = 90 – 20
6θ = 90
`θ = 90/6`
θ = 15°
APPEARS IN
संबंधित प्रश्न
In ΔPQR, right angled at Q, PQ = 4 cm and RQ = 3 cm. Find the values of sin P, sin R, sec P and sec R.
f θ = 30°, verify that cos 3θ = 4 cos3 θ − 3 cos θ
If cos θ = `7/25` find the value of all T-ratios of θ .
In ΔABC , ∠C = 90° ∠ABC = θ° BC = 21 units . and AB= 29 units. Show thaT `(cos^2 theta - sin^2 theta)=41/841`
From the following figure, find the values of
(i) cos A
(ii) cosec A
(iii) tan2A - sec2A
(iv) sin C
(v) sec C
(vi) cot2 C - ` 1 / sin^2 "c"`

In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sinB = `sqrt(3)/(2)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sec B = `(15)/(12)`
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: sin P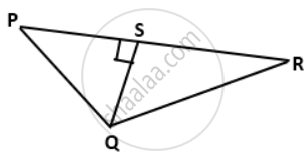
If sin A = `(7)/(25)`, find the value of : cot2A - cosec2A
If cosec θ = `(29)/(20)`, find the value of: `("sec" θ)/("tan" θ - "cosec" θ)`
