Advertisements
Advertisements
प्रश्न
If cos θ : sin θ = 1 : 2, then find the value of `(8costheta - 2sintheta)/(4costheta + 2sintheta`
उत्तर
cos θ : sin θ = 1 : 2
The value of `(8costheta - 2sintheta)/(4costheta + 2sintheta`
= `(8(sintheta/2) - 2sintheta)/(4(sintheta/2) + 2sintheta)`
= `(4 sintheta - 2 sintheta)/(2 sintheta + 2 sintheta)`
= `(2 sintheta)/(4 sintheta)`
= `2/4`
= `1/2`
Aliter:
cos θ : sin θ = 1 : 2
cos θ = sin θ
⇒ 2 = `sintheta/costheta`
⇒ 2 = tan θ
AC = `sqrt(1^2 + 2^2)`
= `sqrt(1 + 4)`
⇒ `sqrt(5)`
∴ sin θ = `2/sqrt(5)`, cos θ = `1/sqrt(5)`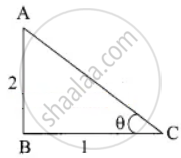
The value of `(8 cos theta - 2 sin theta)/(4 cos theta + 2 sin theta)`
= `8(1/sqrt(5)) - 2(2/sqrt(5)) ÷ 4(1/sqrt(5)) + 2 xx (2/sqrt(5))`
= `(8/sqrt(5)) - (4/sqrt(5)) ÷ (4/sqrt(5)) + (4/sqrt(5))`
= `((8 - 4)/sqrt(5)) ÷ ((4 + 4)/sqrt(5))`
= `(4/sqrt(5)) ÷ (8/sqrt(5))`
= `(4/sqrt(5)) xx (sqrt(5)/8)`
= `(4/8)`
= `1/2`
∴ The value is `1/2`
APPEARS IN
संबंधित प्रश्न
if `cos theta = 5/13` find the value of `(sin^2 theta - cos^2 theta)/(2 sin theta cos theta) = 3/5`
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
If a right ΔABC , right-angled at B, if tan A=1 then verify that 2sin A . cos A = 1
Evaluate:
`4/(cot^2 30^0) +1/(sin^2 30^0) -2 cos^2 45^0 - sin^2 0^0`
Verify each of the following:
(i)`sin 60^0 cos 30^0-cos 60^0 sin 30^0`
If A = 300 , verify that:
(iii) tan 2A = `(2tanA)/(1-tan^2A)`
Using the measurements given in the following figure:
(i) Find the value of sin θ and tan θ.
(ii) Write an expression for AD in terms of θ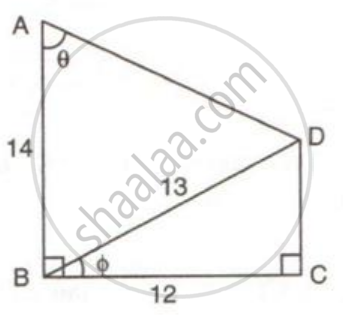
Given : 5 cos A - 12 sin A = 0; evaluate:
`(sin "A"+cos"A")/(2 cos"A"– sin"A")`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`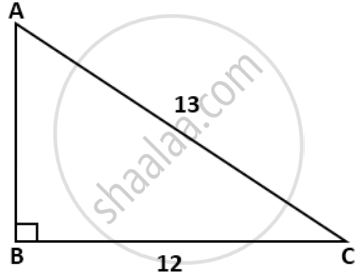
If cos A = `(2x)/(1 + x^2)`, then find the values of sin A and tan A in terms of x
