Advertisements
Advertisements
प्रश्न
Given : 5 cos A - 12 sin A = 0; evaluate:
`(sin "A"+cos"A")/(2 cos"A"– sin"A")`
उत्तर
5 cos A – 12 sin A = 0
5 cos A = 12 sin A
`sin "A"/cos "A" = (5)/(12)`
tan A = `(5)/(12)`
Now,
`(sin "A"+cos"A")/(2 cos"A"– sin"A") = (sin"A"/cos"A" + cos"A"/cos"A")/(2 cos"A"/cos"A" – sin"A"/cos"A")`
= `(tan "A"+1)/(2– tan "A")`
= `(5/12+1)/(2–5/12)`
= `(17/12)/(19/12)`
= `(17)/(19)`
APPEARS IN
संबंधित प्रश्न
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
Evaluate:
`2cos^2 60^0+3 sin^2 45^0 - 3 sin^2 30^0 + 2 cos^2 90 ^0`
Verify each of the following:
(ii)`cos 60^0 cos 30^0+ sin 60^0 sin30^0`
`(cos 28°)/(sin 62°)` = ?
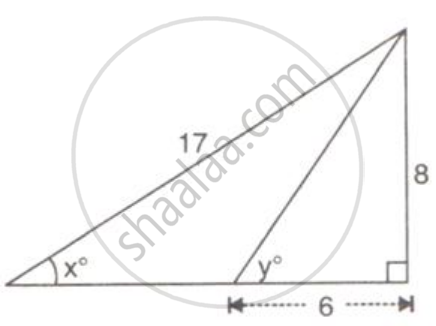
Use the given figure to find :
(i) sin xo
(ii) cos yo
(iii) 3 tan xo - 2 sin yo + 4 cos yo.
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: sinB
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: sinA
If cos A = `3/5`, then find the value of `(sin"A" - cos"A")/(2tan"A")`
If A + B = 90°, cot B = `3/4` then tan A is equal to ______.
