Advertisements
Advertisements
प्रश्न
If cos A = `3/5`, then find the value of `(sin"A" - cos"A")/(2tan"A")`
उत्तर

cos A = `3/5`
ΔABC
BC2 = AC2 – AB2
= 52 – 32
= 25 – 9
= 16
BC = `sqrt(16)` = 4
sin A = `4/5`, tan A = `4/3`
`(sin"A" - cos"A")/(2tan"A") = 4/5 - 3/5 ÷ 2(4/3)`
= `(4 - 3)/5 ÷(8/3)`
= `1/5 xx (3/8)`
= `3/40`
∴ The value of `(sin"A" - cos"A")/(2tan"A") = 3/40`
APPEARS IN
संबंधित प्रश्न
If A = 30° B = 60° verify Sin (A + B) = Sin A Cos B + cos A sin B
If 3 cot `theta = 2, `show that `((4 sin theta - 4 cos theta))/((2 sin theta + 6 cos theta ))=1/3`
Verify each of the following:
(ii)`cos 60^0 cos 30^0+ sin 60^0 sin30^0`
Using the formula, cos A = `sqrt((1+cos2A)/2) ,`find the value of cos 300, it being given that cos 600 = `1/2`.
From the following figure, find the values of :
(i) sin A
(ii) sec A
(iii) cos2 A + sin2A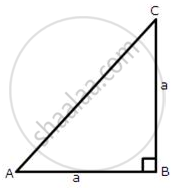
In triangle ABC, AB = AC = 15 cm and BC = 18 cm, find cos ∠ABC.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cotA = `(1)/(11)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
sec B = `(15)/(12)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cosec C = `sqrt(10)`
From the given figure, find all the trigonometric ratios of angle B
