Advertisements
Advertisements
प्रश्न
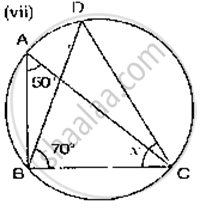
If O is the centre of the circle, find the value of x in the following figure
उत्तर
We have
∠ DBO = 40°
∠ DBC = 90°
⇒ ∠ DBO + ∠OBC = 90°
⇒ 40° + ∠OBC = 90°
⇒ ∠ OBC = 90° - 40° = 50°
By degree measure theorem
ÐAOC = 2ÐOBC
⇒ x = 2´ 50° = 100°
APPEARS IN
संबंधित प्रश्न
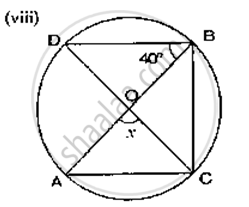
If O is the centre of the circle, find the value of x in the following figure
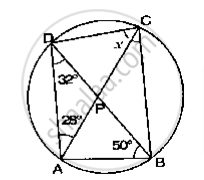
If O is the centre of the circle, find the value of x in the following figure
O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
Prove that the line segment joining the mid-point of the hypotenuse of a right triangle to its opposite vertex is half the hypotenuse.
In the given figure, two circles intersect at A and B. The centre of the smaller circle is Oand it lies on the circumference of the larger circle. If ∠APB = 70°, find ∠ACB.

In the given figure, if ∠AOB = 80° and ∠ABC = 30°, then find ∠CAO.

In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

If ABC is an arc of a circle and ∠ABC = 135°, then the ratio of arc \[\stackrel\frown{ABC}\] to the circumference is ______.
The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
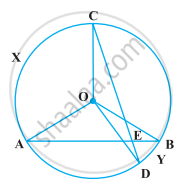
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).