Advertisements
Advertisements
प्रश्न
If the areas of two similar triangles are equal, prove that they are congruent.
उत्तर
Let us assume two similar triangle as ΔABC ~ ΔPQR
`(ar(triangleABC))/(ar(trianglePQR))=((AB)/(PQ))^2 = ((BC)/(QR))^2=((AC)/(PR))^2 ...(1)`
Given that, ar(ΔABC) = ar(ΔABC)
`=>(ar(triangleABC))/(ar(trianglePQR)) =1`
Putting this value in equation (1) we obtain
`1=((AB)/(PQ))^2= ((BC)/(QR))^2=((AC)/(PR))^2`
⇒ AB = PQ, BC = QR and AC = PR
∴ ΔABC ≅ ΔPQR (By SSS congruence criterion)
APPEARS IN
संबंधित प्रश्न
If ∆ABC ~ ∆DEF such that area of ∆ABC is 16cm2 and the area of ∆DEF is 25cm2 and BC = 2.3 cm. Find the length of EF.
D, E, F are the mid-point of the sides BC, CA and AB respectively of a ∆ABC. Determine the ratio of the areas of ∆DEF and ∆ABC.
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
In figure below ΔACB ~ ΔAPQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm,
find CA and AQ. Also, find the area (ΔACB): area (ΔAPQ)

Triangles ABC and DEF are similar If AC = 19cm and DF = 8 cm, find the ratio of the area of two triangles.
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 m, prove that area of ΔAPQ is one- sixteenth of the area of ABC.
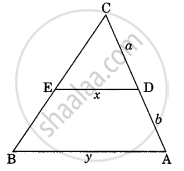
In the given figure DE || AC which of the following is true?
In the given figure, ΔACB ~ ΔAPQ. If AB = 6 cm, BC = 8 cm, and PQ = 4 cm then AQ is equal to ______.

If the perimeter of two similar triangles is in the ratio 2 : 3, what is the ratio of their sides?
If ΔABC ∼ ΔPQR and `(A(ΔABC))/(A(ΔPQR)) = 16/25`, then find AB : PQ.
