Advertisements
Advertisements
प्रश्न
In a ABC , AD is a median and AL ⊥ BC .
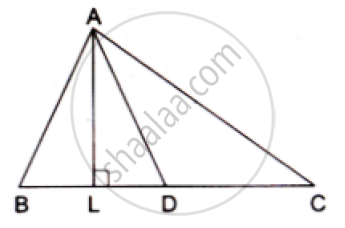
Prove that
(a) `AC^2=AD^2+BC DL+((BC)/2)^2`
(b) `AB^2=AD^2-BC DL+((BC)/2)^2`
(c) `AC^2+AB^2=2.AD^2+1/2BC^2`
उत्तर १
(a) In right triangle ALD
Using Pythagoras theorem, we have
`=AC^2-AL^2+LC^2`
`=AD^2-DL^2+(DL+DC)^2 ` [Using (1)]
=`AD^2-DL^2+(DL+(BC)/2)^2` [∵ AD is a median]
`=AD^2-DL^2+DL^2+((BC)/2)^2+BC.DL `
∴` AC^2=AD^2+BC.DL+((BC)/2)^2` .................(2)
(b) In right triangle ALD
Using Pythagoras theorem, we have
`AL^2=AD^2-DL^2` ....................(3)
Again, In right triangle ABL
Using Pythagoras theorem, we have
`AB^2=AL^2+LB^2`
`=AD^2-DL^2+LB^2` [𝑈𝑠𝑖𝑛𝑔 (3)]
उत्तर २
(a) In right triangle ALD
Using Pythagoras theorem, we have
`=AC^2-AL^2+LC^2`
`=AD^2-DL^2+(DL+DC)^2 ` [Using (1)]
=`AD^2-DL^2+(DL+(BC)/2)^2` [∵ AD is a median]
`=AD^2-DL^2+DL^2+((BC)/2)^2+BC.DL `
∴` AC^2=AD^2+BC.DL+((BC)/2)^2` .................(2)
(b) In right triangle ALD
Using Pythagoras theorem, we have
`AL^2=AD^2-DL^2` ....................(3)
Again, In right triangle ABL
Using Pythagoras theorem, we have
`AB^2=AL^2+LB^2`
`=AD^2-DL^2+LB^2` [𝑈𝑠𝑖𝑛𝑔 (3)]
=`AD^2-DL^2+(BD-DL)^2`
=`AD^2DL^2+(1/2BC-DL)^2`
=`AD^2-DL^2+((BC)/2)^2-BC.DL+DL^2`
∴ AB^2=AD^2-BC.DL+((BC)/2)^2 .............(4)
(c) Adding (2) and (4), we get,
=`AC^2+AB^2=AD^2+BC.DL+((BC)/2)^2+AD^2-BC.DL+((BC)/2)^2`
=`2AD^2+(BC^2)/4+(BC^2)/4`
=`2AD^2+(BC^2)/4+(BC^2)/4`
=`2AD^2+1/2BC^2`
APPEARS IN
संबंधित प्रश्न
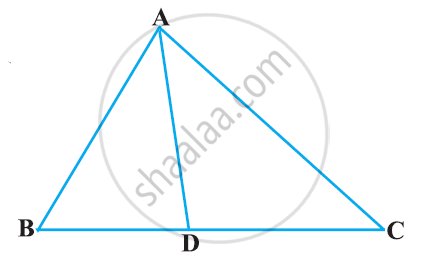
In the given figure, D is a point on side BC of ΔABC such that ∠ADC=∠BAC . Prove that AD is the bisector of ∠BAC.
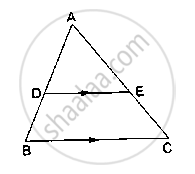
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = x, DB = x − 2, AE = x + 2 and EC = x − 1, find the value of x.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 2cm, AD = 8cm, AE = 12 cm and AC = l8cm.
In a ΔABC, P and Q are points on sides AB and AC respectively, such that PQ || BC. If AP = 2.4 cm, AQ = 2 cm, QC = 3 cm and BC = 6 cm, find AB and PQ.
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If` (AD)/(AB) = 8/15 and EC = 3.5cm`, find AE.
Find the length of a diagonal of a rectangle whose adjacent sides are 30cm and 16cm.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
In the given figure, D is the midpoint of side BC and AE⊥BC. If BC = a, AC = b, AB = c, AD = p and AE = h, prove that
(i)`B^2=p^2+ax+a^2/x`
(ii)` c^2=p^2-ax+a^2/x`
(iii) `b^2+c^2=2p^2+a^2/2`
(iv)`b^2-c^2=2ax`

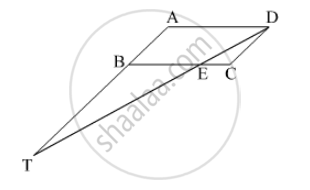
◻ABCD is a parallelogram point E is on side BC. Line DE intersects ray AB in point T. Prove that DE × BE = CE × TE.
A line is parallel to one side of triangle which intersects remaining two sides in two distinct points then that line divides sides in same proportion.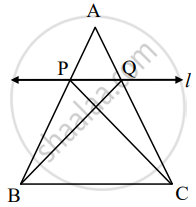
Given: In ΔABC line l || side BC and line l intersect side AB in P and side AC in Q.
To prove: `"AP"/"PB" = "AQ"/"QC"`
Construction: Draw CP and BQ
Proof: ΔAPQ and ΔPQB have equal height.
`("A"(Δ"APQ"))/("A"(Δ"PQB")) = (["______"])/"PB"` .....(i)[areas in proportion of base]
`("A"(Δ"APQ"))/("A"(Δ"PQC")) = (["______"])/"QC"` .......(ii)[areas in proportion of base]
ΔPQC and ΔPQB have [______] is common base.
Seg PQ || Seg BC, hence height of ΔAPQ and ΔPQB.
A(ΔPQC) = A(Δ______) ......(iii)
`("A"(Δ"APQ"))/("A"(Δ"PQB")) = ("A"(Δ "______"))/("A"(Δ "______"))` ......[(i), (ii), and (iii)]
`"AP"/"PB" = "AQ"/"QC"` .......[(i) and (ii)]
