Advertisements
Advertisements
प्रश्न
In ΔABC, MN is drawn parallel to BC. If AB = 3.5cm, AM : AB = 5 : 7 and NC = 2cm, find:
(i) AM
(ii) AC
उत्तर
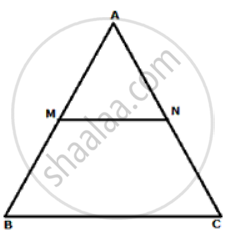
(i) `"AM"/"AB" = (5)/(7)`
∵ AB = 3.5cm
∴ AM = `(5 xx "AB")/(7)`
⇒ AM = `(5 xx 3.5)/(7)`
⇒ AM = 2.5cm.
(ii) Since MN || BC and `"AM"/"MB" = "AN"/"NC"`
∵ AB = 3.5cm; AM = 2.5cm
∴ MB
= AB - AM
= 3.5 - 2.5
= 1cm
⇒ `"AM"/"MB" = "AN"/"NC"`
⇒ `(2.5)/(1) = "AN"/(2)`
⇒ AN = `(2.5 xx 2)/(1)` = 5cm
Now,
AC = AN + NC
⇒ AC
= 5 + 2
= 7cm.
APPEARS IN
संबंधित प्रश्न
Using Converse of basic proportionality theorem, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side. (Recall that you have done it in Class IX).
In the following figure, seg DH ⊥ seg EF and seg GK ⊥ seg EF. If DH = 18 cm, GK = 30 cm and `A(triangle DEF) = 450 cm^2`, then find:
1) EF
2) `A(triangle GFE)`
3) `A(square DFGE)`

In the given figure, ABC is a triangle. DE is parallel to BC and `(AD)/(DB) = 3/2`.
- Determine the ratios `(AD)/(AB)` and `(DE)/(BC)`.
- Prove that ∆DEF is similar to ∆CBF. Hence, find `(EF)/(FB).`
- What is the ratio of the areas of ∆DEF and ∆BFC?
In the given figure, ∠CAB = 90° and AD⊥BC. Show that ΔBDA ~ ΔBAC. If AC = 75cm, AB = 1m and BC = 1.25m, find AD.

In Δ ABC , DE is parallel to BC and `"AD"/"DB" = 2/7` IF AC = 5 .6 , find AE.
In ΔABC, AB = 8cm, AC = 10cm and ∠B = 90°. P and Q are the points on the sides AB and AC respectively such that PQ = 3cm ad ∠PQA = 90. Find: The area of ΔAQP.
From the given figure, prove that ΔABC ~ ΔEDF
Are triangles in figure similar? If yes, then write the test of similarity.
Two triangles are similar. Smaller triangle’s sides are 4 cm, 5 cm, 6 cm. Perimeter of larger triangle is 90 cm then find the sides of larger triangle.
ΔABC and ΔBDE are two equilateral triangles such that D is the mid point of BC. Ratio of the areas of triangle ΔABC and ΔBDE is ______.
