Advertisements
Advertisements
प्रश्न
In fig. BD = 8, BC = 12, B-D-C, then `"A(ΔABC)"/"A(ΔABD)"` = ?
विकल्प
2 : 3
3 : 2
5 : 3
3 : 4
उत्तर
3: 2
In ΔABC and ΔABD,
ΔABC and ΔABD have the same height. ...(Given)
The ratio of the areas of two triangles with equal heights is equal to the ratio of their corresponding bases.
∴ `"A(ΔABC)"/"A(ΔABD)" = "BC"/"BD"`
∴ `"A(ΔABC)"/"A(ΔABD)" = 12/8`
∴ `"A(ΔABC)"/"A(ΔABD)" = 3/2`.
APPEARS IN
संबंधित प्रश्न
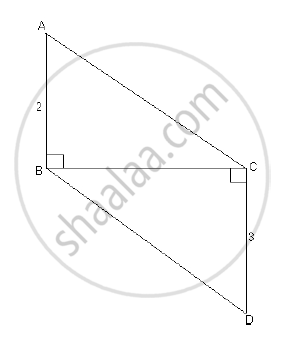
In the following figure seg AB ⊥ seg BC, seg DC ⊥ seg BC. If AB = 2 and DC = 3, find `(A(triangleABC))/(A(triangleDCB))`
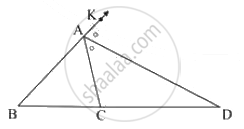
In the following figure RP: PK= 3:2, then find the value of A(ΔTRP):A(ΔTPK).

The ratio of the areas of two triangles with common base is 6:5. Height of the larger triangle of 9 cm, then find the corresponding height of the smaller triangle.
In the given figure, AD is the bisector of the exterior ∠A of ∆ABC. Seg AD intersects the side BC produced in D. Prove that :
Base of a triangle is 9 and height is 5. Base of another triangle is 10 and height is 6. Find the ratio of areas of these triangles.
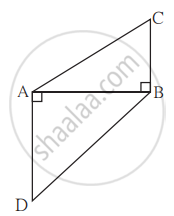
In the given figure, BC ⊥ AB, AD ⊥ AB, BC = 4, AD = 8, then find `("A"(∆"ABC"))/("A"(∆"ADB"))`
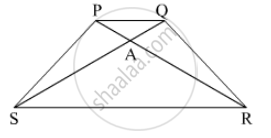
In trapezium PQRS, side PQ || side SR, AR = 5AP, AS = 5AQ then prove that, SR = 5PQ
In ∆ABC, B - D - C and BD = 7, BC = 20 then find following ratio.
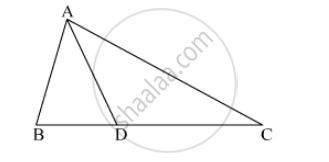
`"A(∆ ABD)"/"A(∆ ADC)"`
Ratio of areas of two triangles with equal heights is 2 : 3. If base of the smaller triangle is 6 cm then what is the corresponding base of the bigger triangle ?
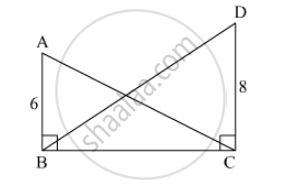
In the given figure, ∠ABC = ∠DCB = 90° AB = 6, DC = 8 then `("A(Δ ABC)")/("A(Δ DCB)")` = ?
The ratio of the areas of two triangles with the common base is 4 : 3. Height of the larger triangle is 2 cm, then find the corresponding height of the smaller triangle.
In ∆ABC, B – D – C and BD = 7, BC = 20 then Find following ratio.

\[\frac{A\left( ∆ ADC \right)}{A\left( ∆ ABC \right)}\]
Areas of two similar triangles are in the ratio 144: 49. Find the ratio of their corresponding sides.
Ratio of corresponding sides of two similar triangles is 4:7, then find the ratio of their areas = ?
From adjoining figure, ∠ABC = 90°, ∠DCB = 90°, AB = 6, DC = 8, then `("A"(Δ"ABC"))/("A"(Δ"BCD"))` = ?

In ΔABC, B − D − C and BD = 7, BC = 20, then find the following ratio.
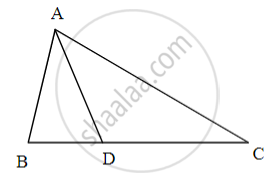
(i) `"A(ΔABD)"/"A(ΔADC)"`
(ii) `"A(ΔABD)"/"A(ΔABC)"`
(iii) `"A(ΔADC)"/"A(ΔABC)"`
Prove that, The areas of two triangles with the same height are in proportion to their corresponding bases. To prove this theorem start as follows:
- Draw two triangles, give the names of all points, and show heights.
- Write 'Given' and 'To prove' from the figure drawn.
If ΔABC ∼ ΔDEF, length of side AB is 9 cm and length of side DE is 12 cm, then find the ratio of their corresponding areas.
