Advertisements
Advertisements
प्रश्न
In fig common tangents PQ and RS to two circles intersect at A. Prove that PQ = RS.
उत्तर
Consider
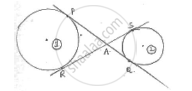
Two circles namely (i) & (ii) as shown with common tangents as PQ and RS.
We know that
The tangents from external point to the circle are equal in length.
From A to circle (i) AP = AR … (i)
From A to circle (ii), AQ = AS …. (ii)
(i) + (ii) ⇒ AP + AQ = AR + RS
⇒ PQ = RS
APPEARS IN
संबंधित प्रश्न
In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively. Prove that AF + BD + CE = AE + CD + BF = `\frac { 1 }{ 2 } ("perimeter of ∆ABC")`
Write True or False. Give reasons for your answers.
A chord of a circle, which is twice as long as its radius, is a diameter of the circle.
Prove that two different circles cannot intersect each other at more than two points.
Two concentric circles are of radii 6.5 cm and 2.5 cm. Find the length of the chord of the larger circle which touches the smaller circle.
In the adjoining figure, a circle touches all the four sides of a quadrilateral ABCD whose sides are AB=6cm, BC=9cm and CD=8 cm. Find the length of side AD.

In the given figure, OQ : PQ = 3.4 and perimeter of Δ POQ = 60 cm. Determine PQ, QR and OP.

The circle which passes through all the vertices of a triangle is called ______.
Construct a triangle PQR in which, PQ = QR = RP = 5.7 cm. Draw the incircle of the triangle and measure its radius.
Find the diameter of the circle
Radius = 10 cm
The circumcentre of a triangle is the point which is ______.
