Advertisements
Advertisements
प्रश्न
In the given figure, OQ : PQ = 3.4 and perimeter of Δ POQ = 60 cm. Determine PQ, QR and OP.

उत्तर
In the figure,
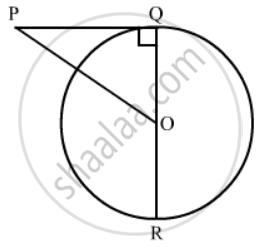
∠ PQD. Therefore we can use Pythagoras theorem to find the side PO.
`PO^2=PQ^2+OQ^2` …… (1)
In the problem it is given that,
`(OQ)/(PQ)=3/4`
`OQ= 3/4PQ`.....(2)
Substituting this in equation (1), we have,
`PQ^2=(9PQ^2)/16+PQ^2`
`PQ^2=(25PQ^2)/16`
`PQ^2=sqrt((25PQ^2)/16)`
`PQ=5/4PQ`........(3)
It is given that the perimeter of Δ POQis 60 cm. Therefore,
PQ + OQ + PO = 60
Substituting (2) and (3) in the above equation, we have,
`PQ+3/4PQ+5/4PQ=60`
`12/4PQ=60`
`3PQ=60`
`PQ=20`
Substituting for PQ in equation (2), we have,
`PD=5/4xx15`
`OQ=3/4xx20`
`OQ=15`
OQ is the radius of the circle and QR is the diameter. Therefore,
QR = 2OQ
QR = 30
Substituting for PQ in equation (3), we have,
`PD=5/4xx20`
`PO=25`
Thus we have found that PQ = 20 cm, QR = 30 cm and PO = 25 cm.
संबंधित प्रश्न
From a point T outside a circle of centre O, tangents TP and TQ are drawn to the circle. Prove that OT is the right bisector of line segment PQ.
In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP

Fill in the blanks:
The centre of a circle lies in ____________ of the circle.
If from any point on the common chord of two intersecting circles, tangents be drawn to circles, prove that they are equal.
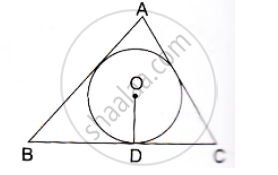
In the fig. ABC is right triangle right angled at B such that BC = 6cm and AB = 8cm. Find the radius of its in circle.
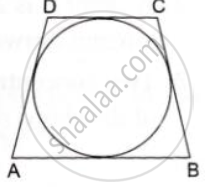
In fig. a circle touches all the four sides of quadrilateral ABCD with AB = 6cm, BC = 7cm, CD = 4cm. Find AD.
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
In the given figure, a circle touches all the four sides of a quadrilateral ABCD whose three sides are AB = 6cm, BC=7cm and CD=4 cm. Find AD.
A circle is inscribed in a ΔABC touching AB, BC and AC at P, Q and R respectively. If AB = 10 cm, AR=7cm and CR=5cm, find the length of BC.

In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 2 cm such that the segments BD and DC into which BC is divided by the point of contact D, are of lengths 4cm and 3cm respectively. If the area of 2 ABC 21cm then find the lengths of sides AB and AC.
In the given figure, O is the centre of the circle. PA and PB are tangents. Show that AOBP is cyclic quadrilateral.

If the area of a circle is equal to sum of the areas of two circles of diameters 10 cm and 24 cm, then the diameter of the larger circle (in cm) is:
If ABCD is a cyclic quadrilateral in which AD || BC (In the given figure). Prove that ∠B = ∠C.

If AB, BC and CD are equal chords of a circle with O as centre and AD diameter, than ∠AOB =
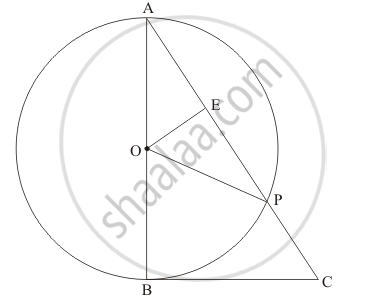
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO ∼ Δ ABC.
Radius of a circle with centre O is 4 cm. If l(OP) = 4.2 cm, say where point P will lie.
Find the length of the chord of a circle in the following when:
Radius is 6.5 cm and the distance from the centre is 2.5 cm
If all the sides of a parallelogram touch a circle, show that the parallelogram is a rhombus.
ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
Draw a circle of radius 3.6 cm. In the circle, draw a chord AB = 5 cm. Now shade the minor segment of the circle.
In a circle, AB and CD are two parallel chords with centre O and radius 10 cm such that AB = 16 cm and CD = 12 cm determine the distance between the two chords?
The longest chord of a circle is __________
A part of circumference of a circle is called as _______
Find the missing values in the following table for the circles with radius (r), diameter (d) and Circumference (C).
| radius (r) | diameter (d) | Circumference (C) |
| 1760 cm |
Find the diameter of the circle
Radius = 8 cm
In figure, chords AC and DE intersect at B. If ∠ABE = 108°, m(arc AE) = 95°, find m(arc DC).

If the radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other circle is ______
A line through the point of contact and passing through centre of the circle is known as ______
If a hexagon ABCDEF circumscribe a circle, prove that AB + CD + EF = BC + DE + FA.
Is every diameter of a circle also a chord?
