Question

If the radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other circle is ______.

विकल्प

• 3 cm

• 6 cm

• 9 cm

• 1 cm

Answer 1

If radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other circle is 6 cm.

Explanation:

Let O be the centre of two concentric circles C₁ and C₂, whose radii are r₁ = 4 cm and r₂ = 5 cm.

Now, we draw a chord AC of circle C₂, which touches the circle C₁ at B.

Also, join OB, which is perpendicular to AC.

[∵ Tangent at any point of circle is perpendicular to radius through the point of contact]

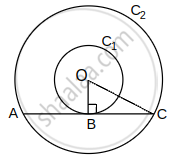
Now, in right angled ∆OBC,

By using Pythagoras theorem,

OC² = BC² + BO²     ...[∵ (Hypotenuse)² = (Base)² + (Perpendicular)²]

⇒ 5² = BC² + 4²

⇒ BC² = 25 – 16 = 9

⇒ BC = 3 cm

∴ Length of chord AC = 2BC = 2 × 3 = 6 cm