Advertisements
Advertisements
प्रश्न
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
उत्तर
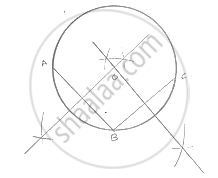
Steps of constructions:
(1) Take three point A, B and C the given circle
(2) Join AB and BC
(3) Draw the perpendicular bisectors of chord AB and BC which intersect each other at O.
(4) Point O will be the required center of the circle because we know that the perpendicular
bisector of the cord always passes through the center
APPEARS IN
संबंधित प्रश्न
In Fig. 1, QR is a common tangent to the given circles, touching externally at the point T. The tangent at T meets QR at P. If PT = 3.8 cm, then the length of QR (in cm) is :
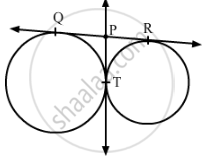
(A) 3.8
(B) 7.6
(C) 5.7
(D) 1.9
Two circles touch externally at a point P. from a point T on the tangent at P, tangents TQ and TR are drawn to the circles with points of contact Q and E respectively. Prove that TQ = TR.
In the given figure, PA and PB are two tangents to the circle with centre O. If ∠APB = 60° then find the measure of ∠OAB.

The length of three concesutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm, and 7 cm respectively. Determine the length of the fourth side.
In an equilateral triangle, prove that the centroid and center of the circum-circle (circumcentre) coincide.
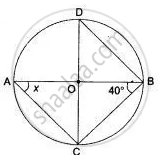
If O is the centre of the circle, find the value of x in each of the following figures
In the figure, O is the center of the circle. Line AQ is a tangent. If OP = 3, m(arc PM) = 120°, then find the length of AP.
If a chord AB subtends an angle of 60° at the centre of a circle, then angle between the tangents at A and B is also 60°.
From the figure, identify a chord.

Is every chord of a circle also a diameter?
