Advertisements
Advertisements
Question
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
Solution
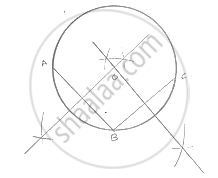
Steps of constructions:
(1) Take three point A, B and C the given circle
(2) Join AB and BC
(3) Draw the perpendicular bisectors of chord AB and BC which intersect each other at O.
(4) Point O will be the required center of the circle because we know that the perpendicular
bisector of the cord always passes through the center
APPEARS IN
RELATED QUESTIONS
Prove that the tangents at the extremities of any chord make equal angles with the chord.
In the given figure, two tangents RQ, and RP and RP are drawn from an external point R to the circle with centre O. If ∠PRQ =120° , then prove that OR = PR + RQ.
The perimeter (in cm) of a square circumscribing a circle of radius a cm, is
The greatest chord of a circle is called its
Use the figure given below to fill in the blank:
______ is a chord of the circle.

State, if the following statement is true or false:
If the end points A and B of the line segment lie on the circumference of a circle, AB is a diameter.
The diameter of the circle is 52 cm and the length of one of its chord is 20 cm. Find the distance of the chord from the centre
If a chord AB subtends an angle of 60° at the centre of a circle, then the angle between the tangents at A and B is ______
If an isosceles triangle ABC, in which AB = AC = 6 cm, is inscribed in a circle of radius 9 cm, find the area of the triangle.
From the figure, identify a diameter.

