Advertisements
Advertisements
Question
If an isosceles triangle ABC, in which AB = AC = 6 cm, is inscribed in a circle of radius 9 cm, find the area of the triangle.
Solution
Join OB, OC and OA.
In ∆ABO and ∆ACO,
AB = AC ...[Given]
BO = CO ...[Radii of same circle]
AO = AO ...[Common side]
∴ ∆ABO ≅ ∆ACO ...[By SSS congruence criterion]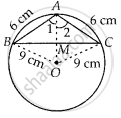
⇒ ∠1 = ∠2 ...[CPCT]
Now, In ∆ABM and ∆ACM,
AB = AC ...[Given]
∠1 = ∠2 ...[Proved above]
AM = AM ...[Common side]
∴ ∆AMB ≅ ∆AMC ...[By SAS congruence criterion]
⇒ ∠AMB = ∠AMC ...[CPCT]
Also, ∠AMB + ∠AMC = 180° ...[Linear pair]
⇒ ∠AMB = 90°
We know that a perpendicular from the centre of circle bisects the chord.
So, OA is a perpendicular bisector of BC.
Let AM = x, then OM = 9 – x ...[∵ OA = radius = 9 cm]
In right angle ∆AMC,
AC2 = AM2 + MC2 ...[By Pythagoras theorem]
⇒ MC2 = 62 – x2 …(i)
In right angle ∆OMC,
OC2 = OM2 + MC2 ...[By Pythagoras theorem]
⇒ MC2 = 92 – (9 – x)2
From equation (i) and (ii),
62 – x2 = 92 – (9 – x)2
⇒ 36 – x2 = 81 – (81 + x2 – 18x)
⇒ 36 = 18x
⇒ x = 2
∴ AM = 2 cm
From equation (ii),
MC2 = 92 – (9 – 2)2
⇒ MC2 = 81 – 49 = 32
⇒ MC = `4sqrt(2)` cm
∴ BC = 2 MC = `8sqrt(2)` cm
∴ Area of ∆ABC = `1/2` × Base × Height
= `1/2 xx "BC" xx "AM"`
= `1/2 xx 8sqrt(2) xx 2`
= `8sqrt(2) "cm"^2`
Hence, the required area of ∆ABC is `8sqrt(2) "cm"^2`.
APPEARS IN
RELATED QUESTIONS
A circle touches the side BC of a ΔABC at a point P and touches AB and AC when produced at Q and R respectively. As shown in the figure that AQ = `1/2` (Perimeter of ΔABC).

O is the center of a circle of radius 8cm. The tangent at a point A on the circle cuts a line through O at B such that AB = 15 cm. Find OB
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 2 cm such that the segments BD and DC into which BC is divided by the point of contact D, are of lengths 4cm and 3cm respectively. If the area of 2 ABC 21cm then find the lengths of sides AB and AC.
In the given figure, if chords AB and CD of the circle intersect each other at right angles, then x + y =
In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

In the given figure, chord EF || chord GH. Prove that, chord EG ≅ chord FH. Fill in the blanks and write the proof.

Use the figure given below to fill in the blank:
R is the _______ of the circle.

Use the figure given below to fill in the blank:
AB is a ______ of the circle.

In the figure, O is the centre of a circle, AB is a chord, and AT is the tangent at A. If ∠AOB = 100°, then ∠BAT is equal to ______

From the figure, identify a chord.

