Advertisements
Advertisements
प्रश्न
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 2 cm such that the segments BD and DC into which BC is divided by the point of contact D, are of lengths 4cm and 3cm respectively. If the area of 2 ABC 21cm then find the lengths of sides AB and AC.
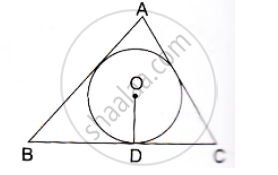
उत्तर
Construction: Join OA, OB, OC, OE ⊥ AB at E and OF ⊥ AC at F
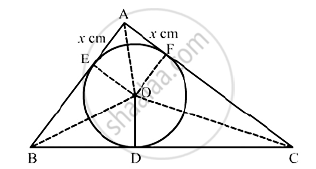
We know that tangent segments to a circle from the same external point are congruent
Now, we have
AE = AF,BD = BE = 4cmand CD = CF = 3cm
Now,
Area (ΔABC) = Area(ΔBOC)+ Area(ΔAOB) + Area(ΔAOC)
`⇒21 = 1/2 xx BC xx OD +1/2 xx AB xx OE +1/2 xx AC xx OF`
`⇒ 42 = 7 xx 2 + (4+x) xx 2 +(3+ x) xx2`
⇒ 21 = 7 + 4 + x + 3 + x
⇒ 21=14+2x
⇒ 2x =7
⇒ x= 3.5 cm
∴ AB = 4 + 3.5 = 7.5 cm and AC = 3 +3.5 = 6.5 cm
APPEARS IN
संबंधित प्रश्न
In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively. Prove that AF + BD + CE = AE + CD + BF = `\frac { 1 }{ 2 } ("perimeter of ∆ABC")`
Prove that there is one and only one tangent at any point on the circumference of a circle.
If ΔABC is isosceles with AB = AC and C (0, 2) is the in circle of the ΔABC touching BC at L, prove that L, bisects BC.
In the fig. a circle is inscribed in a quadrilateral ABCD in which ∠B = 90° if AD = 23cm,
AB = 29cm and DS = 5cm, find the radius of the circle.
In the given figure, PA and PB are two tangents to the circle with centre O. If ∠APB = 60° then find the measure of ∠OAB.

In the given figure, if ABC is an equilateral triangle. Find ∠BDC and ∠BEC.
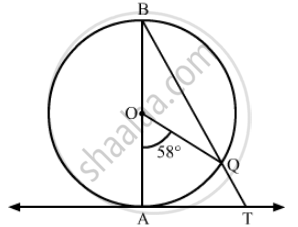
In the given figure, AB is a diameter of a circle with centre O and AT is a tangent. If \[\angle\] AOQ = 58º, find \[\angle\] ATQ.
Find the length of the chord of a circle in the following when:
Radius is 1. 7cm and the distance from the centre is 1.5 cm
Find the diameter of the circle
Radius = 6 cm
On a common hypotenuse AB, two right triangles ACB and ADB are situated on opposite sides. Prove that ∠BAC = ∠BDC.
