Advertisements
Advertisements
प्रश्न
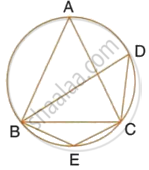
In the figure, m∠DBC = 58°. BD is the diameter of the circle. Calculate:
1) m∠BDC
2) m∠BEC
3) m∠BAC

उत्तर
1) Given that BD is a diameter of the circle
The angle in a semicircle is a right angle.
∴ ∠BCD = 90°
In ΔBDC, by angle sum property, we have
∠DBC + ∠BCD + ∠BDC = 180°
⇒ 58° + 90° + ∠BDC = 180°
⇒ 148° + ∠BDC = 180°
⇒ ∠BDC = 180° - 148°
⇒ ∠BDC = 32°
2) quadrilateral BECD is a cyclic quadrilateral
⇒ ∠BEC + ∠BCD = 180° (Opposite angles are supplementary)
⇒ ∠BEC + 32° = 180°
⇒ ∠BEC = 148°
3) Angles in the same segment are equal.
⇒ ∠BAC = ∠BDC = 32°
APPEARS IN
संबंधित प्रश्न
Prove that the parallelogram, inscribed in a circle, is a rectangle.
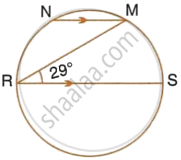
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠RNM
In the figure, given alongside, AB || CD and O is the centre of the circle. If ∠ADC = 25°; find the angle AEB. Give reasons in support of your answer.

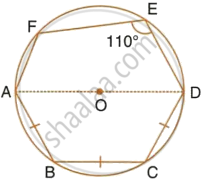
In the following figure, AD is the diameter of the circle with centre O. Chords AB, BC and CD are equal. If ∠DEF = 110°, calculate: ∠AEF
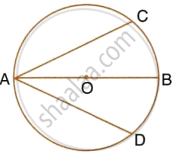
In the given figure, AB is the diameter of a circle with centre O.
If chord AC = chord AD, prove that:
- arc BC = arc DB
- AB is bisector of ∠CAD.
Further, if the length of arc AC is twice the length of arc BC, find:
- ∠BAC
- ∠ABC
Using ruler and a compass only construct a semi-circle with diameter BC = 7cm. Locate a point A on the circumference of the semicircle such that A is equidistant from B and C. Complete the cyclic quadrilateral ABCD, such that D is equidistant from AB and BC. Measure ∠ADC and write it down.
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠DBC
Also, show that the ΔAOD is an equilateral triangle.

In Fig, Chord ED is parallel to the diameter AC of the circle. Given ∠CBE = 65°, Calculate ∠ DEC.
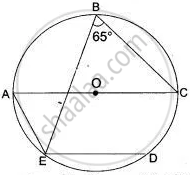
In the figure, ∠DBC = 58°. BD is diameter of the circle.
Calculate:
- ∠BDC
- ∠BEC
- ∠BAC