Advertisements
Advertisements
प्रश्न
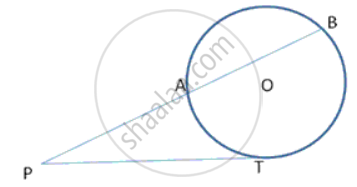
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = Scm, find PT.
उत्तर
Let PT = x cm
Since, PAB is a secant and PT is a tangent to the given circle, we have,
PA · PB = PT2
⇒ 4 · 9 = PT2
⇒ PT2 = 36
⇒ PT = 6cm
APPEARS IN
संबंधित प्रश्न
A circle can have ______ parallel tangents at the most.
A circle touches all the four sides of a quadrilateral ABCD. Prove that AB + CD = BC + DA.
Two chords AB and CD of lengths 6cm and 12cm are drawn parallel inside the circle. If the distance between the chords of the circle is 3cm, find the radius of the circle.
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle APB

In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that - ∠ BAM = ∠ BMA
Draw a circle of radius 2.7 cm and draw a chord PQ of length 4.5 cm. Draw tangents at points P and Q without using centre.
The quadrilateral formed by joining the angle bisectors of a cyclic quadrilateral is a ______
In Question 5 above, if radii of the two circles are equal, prove that AB = CD.
The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
In the given figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 25°, then x is equal to ______.

