Advertisements
Advertisements
प्रश्न
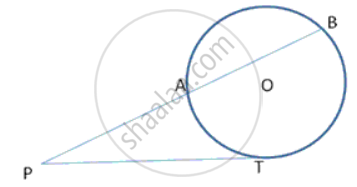
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = Scm, find PT.
उत्तर
Let PT = x cm
Since, PAB is a secant and PT is a tangent to the given circle, we have,
PA · PB = PT2
⇒ 4 · 9 = PT2
⇒ PT2 = 36
⇒ PT = 6cm
APPEARS IN
संबंधित प्रश्न
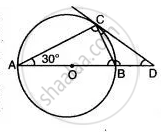
In Fig. 1, PQ is a tangent at a point C to a circle with centre O. if AB is a diameter and ∠CAB = 30°, find ∠PCA.

How many tangents can a circle have?
A line intersecting a circle in two points is called a ______.
In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of a circle.

In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that - ALOB is a cyclic quadrilateral.
In the following figure, seg AB is a diameter of the circle, m (arc AKC) = 40°. Find the value of m (arc BMC).

In Figure, AB is diameter and AC is a chord of a circle such that ∠BAC = 30°. The tangent at C intersects AB produced at D. Prove that BC = BD.
In the figure PA and PB are tangents to the circle with centre O. If ∠APB = 60°, then ∠OAB is ______
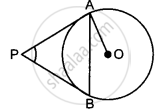
Prove that the tangents drawn at the ends of a chord of a circle make equal angles with the chord.
Construct a pair of tangents to a circle of radius 5 cm which are inclined to each other at an angle of 60°.
