Advertisements
Advertisements
प्रश्न
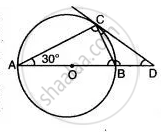
In Figure, AB is diameter and AC is a chord of a circle such that ∠BAC = 30°. The tangent at C intersects AB produced at D. Prove that BC = BD.
उत्तर
Join OC.
∠ ACB = 90° ...(Angle of the semicircle)
∠ ABC = 60° ...(Angle Sum property)
∠ CBD = 120° ...(adj to angle CBA 30°)
∠ OCD = 90° ...(tangent)
∠ COB = 60° ...(Angle at the center is equal to twice that of the circumference)
∠ OCB = 60° ...(Angle Sum property)
∠BCD = ∠ OCD - ∠OCB = 90° - 60° = 30°
∠BDC = ∠BCD = 30°
BD = BC
Hence proved.
APPEARS IN
संबंधित प्रश्न
In Figure 1, AP, AQ and BC are tangents to the circle. If AB = 5 cm, AC = 6 cm and BC
= 4 cm, then the length of AP (in cm) is

In Figure 5, a triangle PQR is drawn to circumscribe a circle of radius 6 cm such that the segments QT and TR into which QR is divided by the point of contact T, are of lengths 12 cm and 9 cm respectively. If the area of ΔPQR = 189 cm2, then find the lengths of sides PQ and PR.
A chord PQ of a circle is parallel to the tangent drawn at a point R of the circle. Prove that R bisects the arc PRQ.
If Δ ABC is isosceles with AB = AC and C (O, r) is the incircle of the ΔABC touching BC at L,prove that L bisects BC.
AB is a diameter and AC is a chord of a circle with centre O such that ∠BAC = 30°. The tangent at C intersects extended AB at a point D. Prove that BC = BD.
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
Calculate the length of direct common tangent to two circles of radii 3cm and Bern with their centres 13cm apart.
A point A is 17cm from the centre of the circle. The length of the tangent drawn from A to the circle is 15cm. find the radius of the circle.
PA and PB are tangents from P to the circle with centre O. At M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.

In the above figure, seg AB and seg AD are tangent segments drawn to a circle with centre C from exterior point A, then prove that: ∠A = `1/2` [m(arc BYD) - m(arc BXD)]
