Question

AB is a diameter and AC is a chord of a circle with centre O such that ∠BAC = 30°. The tangent at C intersects extended AB at a point D. Prove that BC = BD.

Answer 1

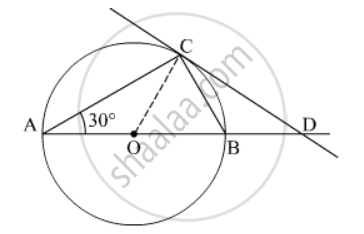
It is given that ∠BAC = 30° and AB is diameter.

`"AB"/2` = OA = OC   ...(Radius)

∠ACB = 90°   ...(Angle formed by the diameter is 90°)

In ∆ABC,

∠ACB + ∠BAC + ∠ABC = 180°

⇒ 90° + 30° + ∠ABC = 180°

⇒ ∠ABC = 60°

⇒ ∠CBD = 180° – 60° = 120°   ...(∠CBD and ∠ABC form a linear pair)

In ∆OCD,

∠OCD = 90°  ...(Angle made by Radius on the tangent)

∠OBC = ∠ABC = 60°

Since OB = OC,

∠OCB = ∠OBC = 60°  ...(OC = OB = radius)

In ∆OCB, 

⇒ ∠COB + ∠OCB + ∠OBC = 180°

⇒ ∠COB + 60° + 60° = 180°

⇒ ∠COB = 60°

In ∆OCD, 

∠COD + ∠OCD  + ∠ODC = 180°

⇒ 60° + 90° + ∠ODC = 90°  ...(∠COD = ∠COB)

⇒ ∠ODC = 30°

In ∆CBD,

∠CBD = 120°

∠BDC = ∠ODC = 30°

⇒ ∠BCD + ∠BDC + ∠CBD = 180°

⇒ ∠BCD + 30° + 120° = 180°

⇒ ∠BCD + 30° = ∠BDC

Angles made by BC and BD on CD are equal, so ∆CBD is an isosceles triangle and therefore, BC = BD.